自律小屋 | 自律小屋

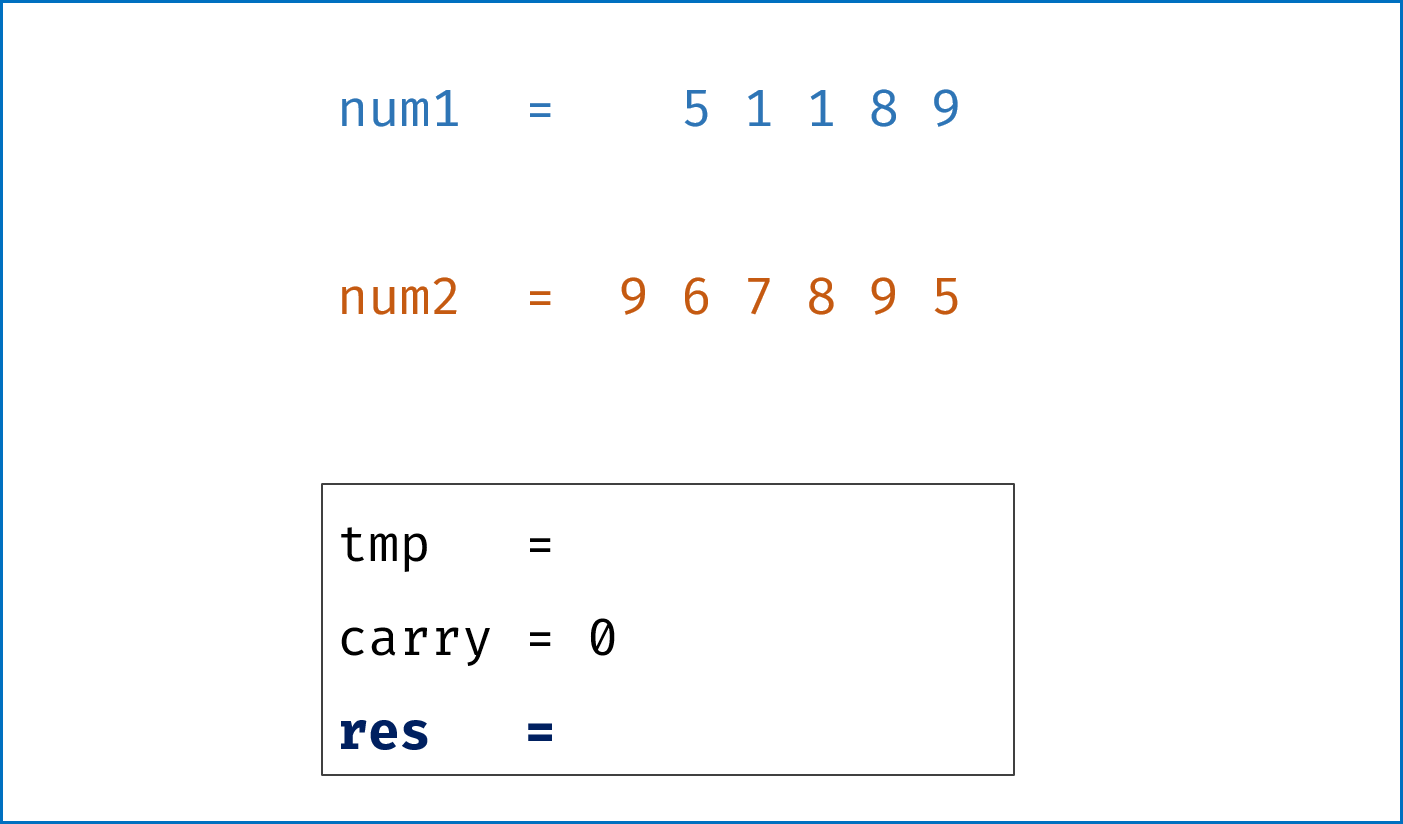
解题思路：

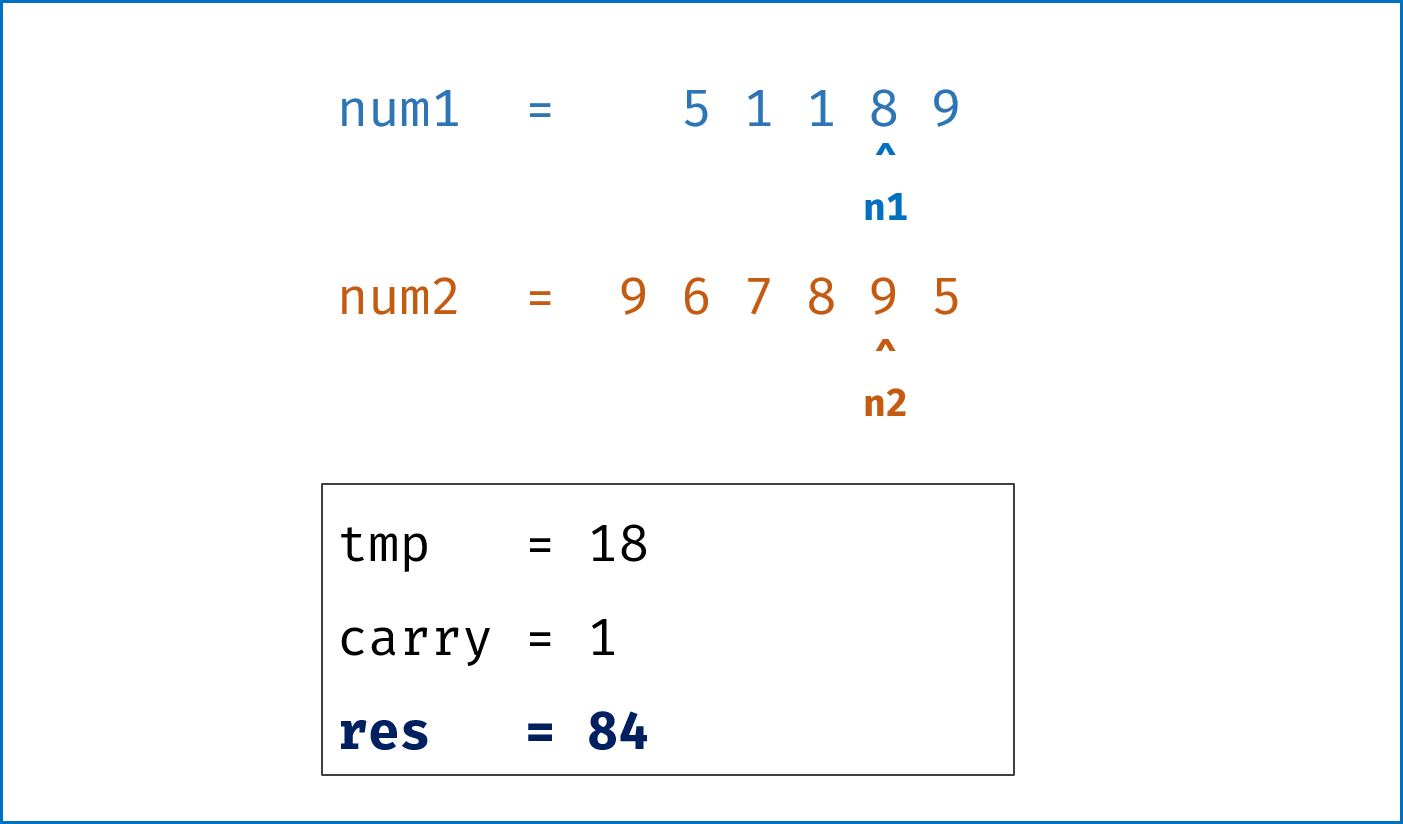
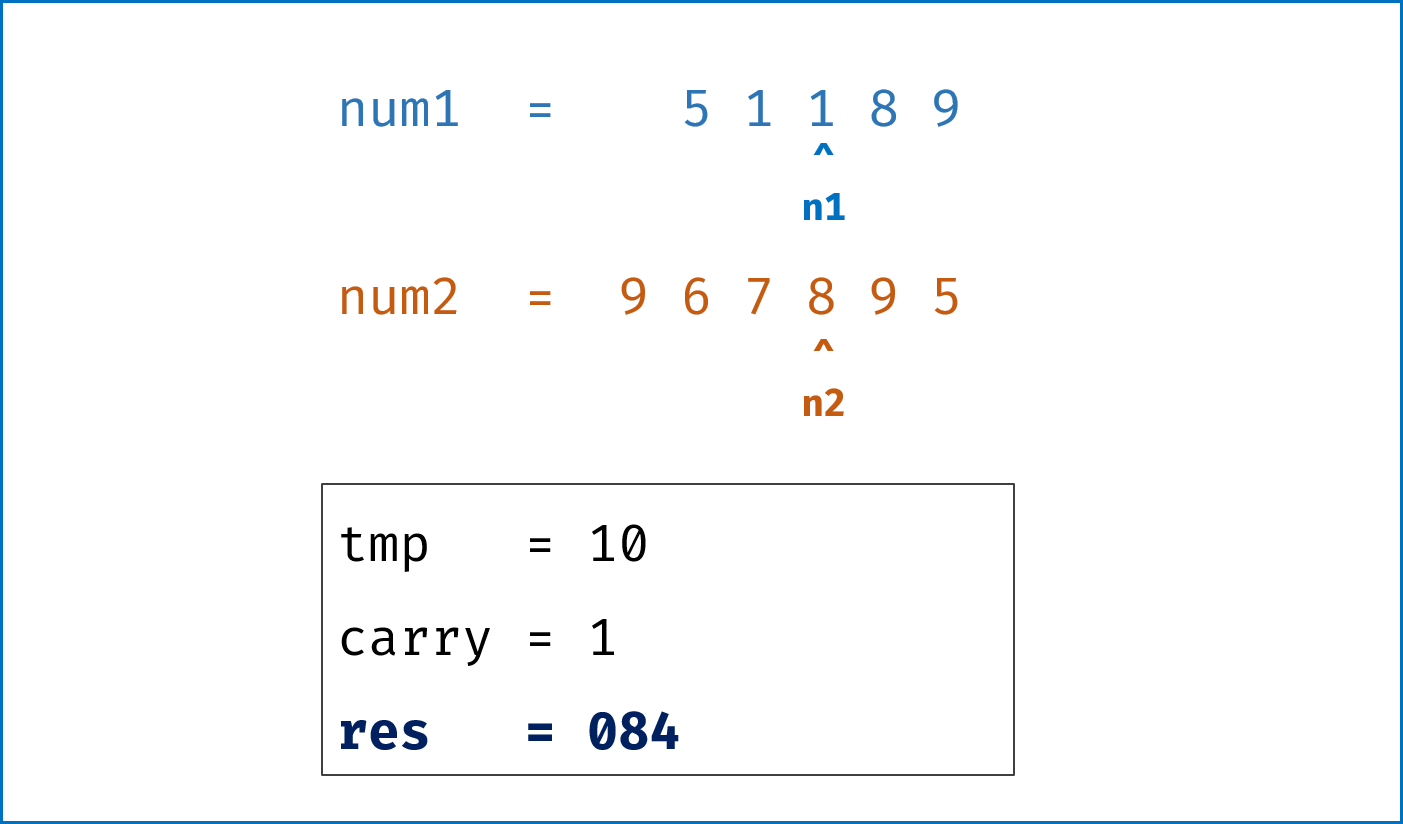
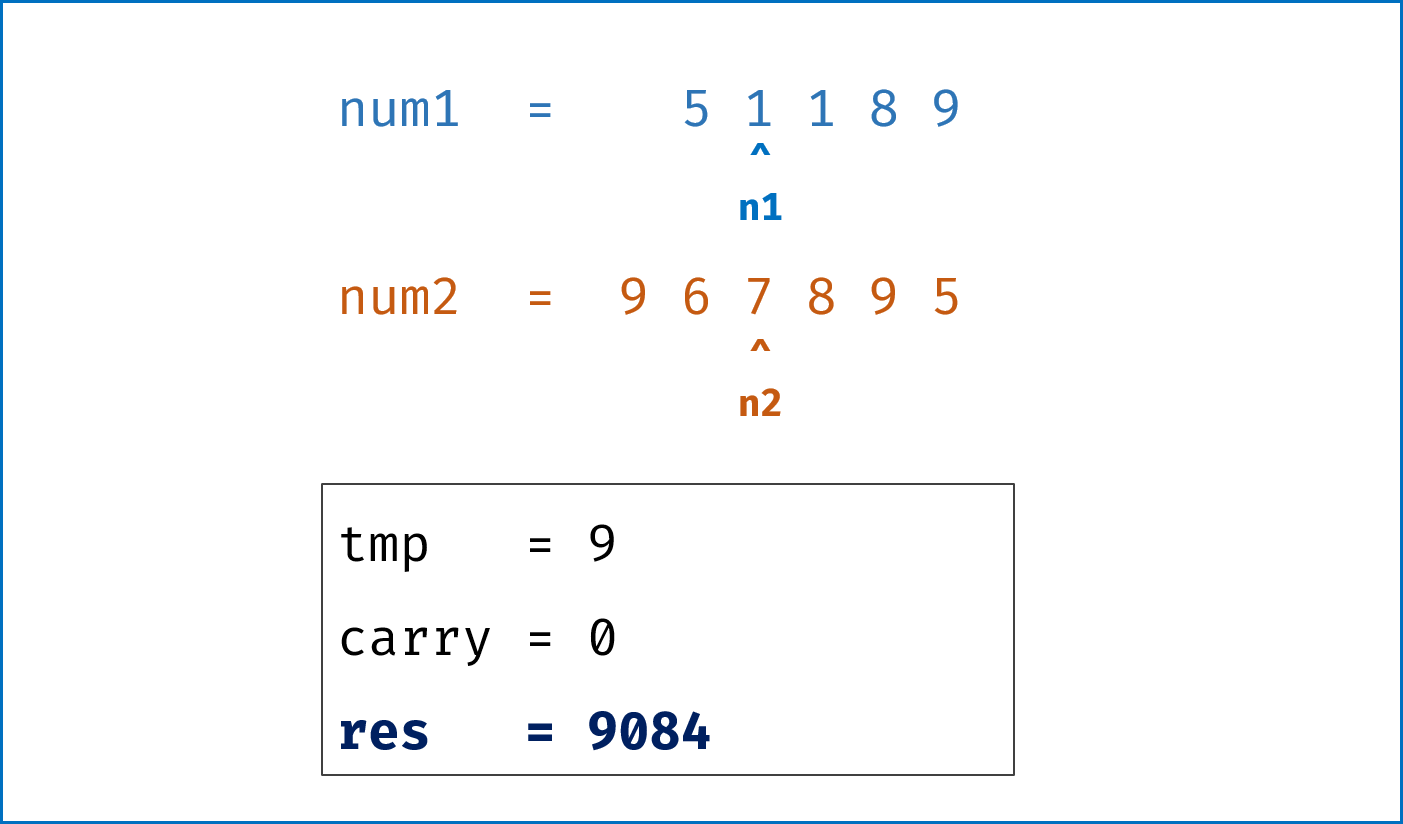
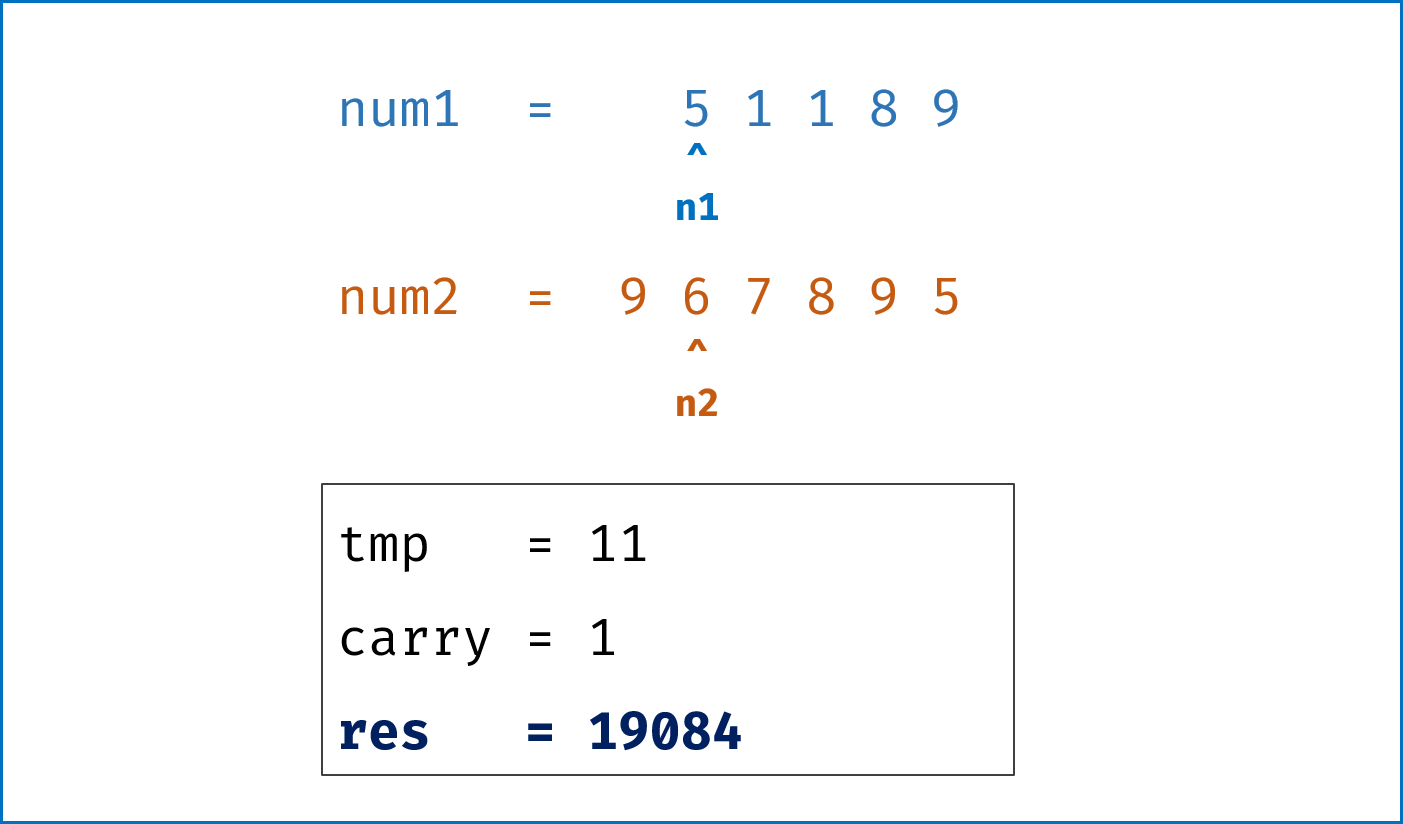
算法流程： 设定 i，j 两指针分别指向 num1，num2 尾部，模拟人工加法；
- 计算进位： 计算 carry = tmp // 10，代表当前位相加是否产生进位；
- 添加当前位： 计算 tmp = n1 + n2 + carry，并将当前位 tmp % 10 添加至 res 头部；
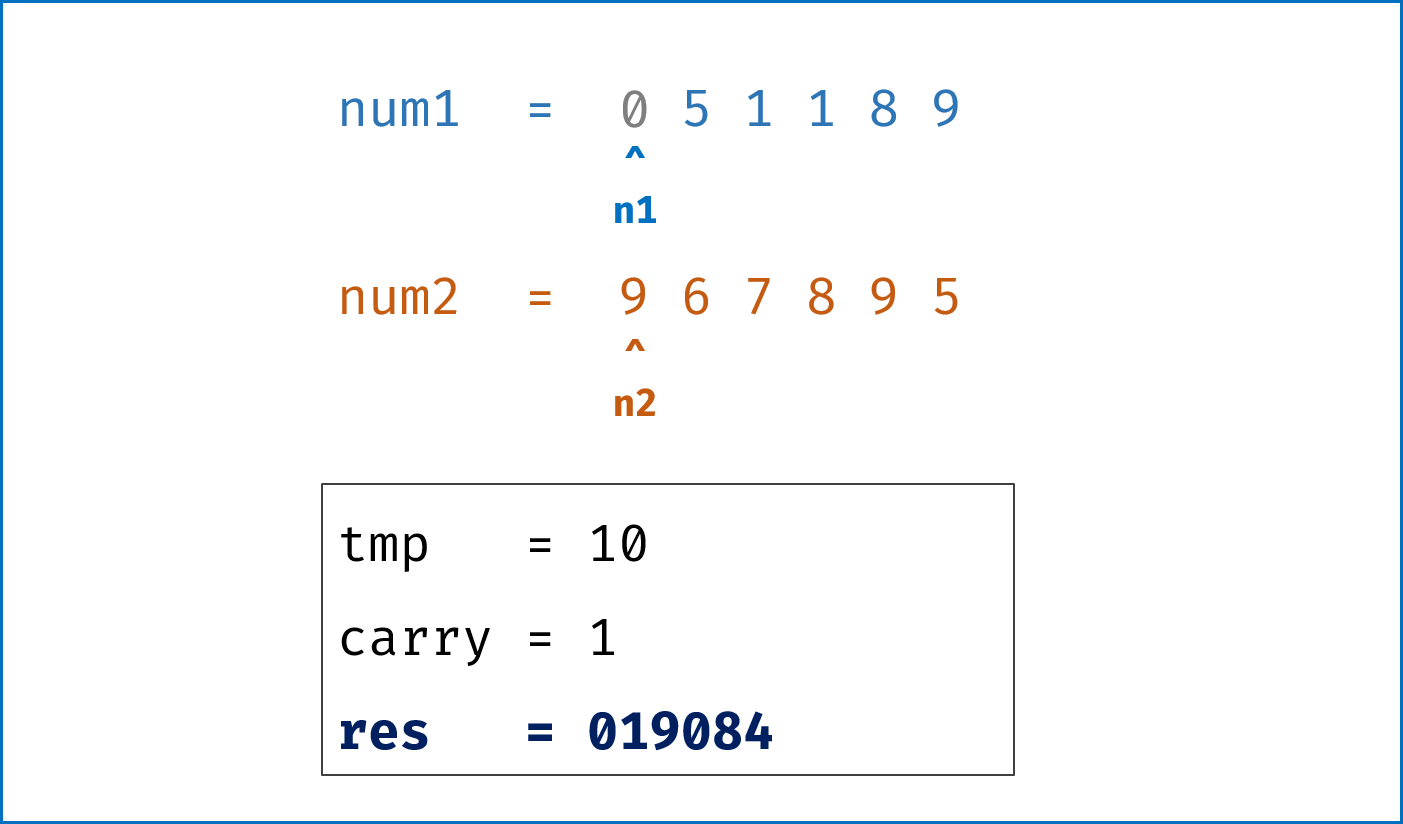
- 索引溢出处理： 当指针 i或j 走过数字首部后，给 n1，n2 赋值为 $0$，相当于给 num1，num2 中长度较短的数字前面填 $0$，以便后续计算。
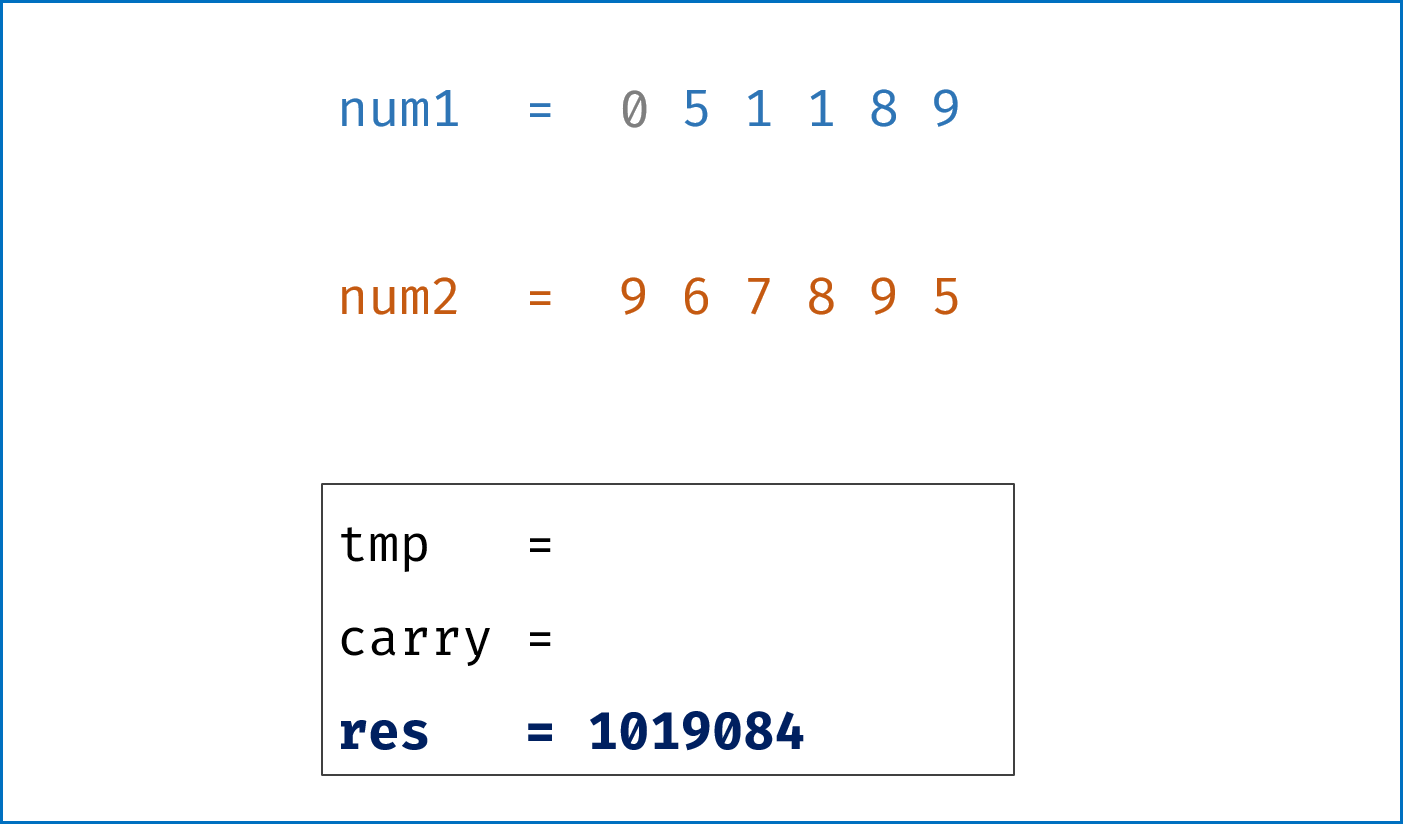
- 当遍历完 num1，num2 后跳出循环，并根据 carry 值决定是否在头部添加进位 $1$，最终返回 res 即可。
复杂度分析：
- 时间复杂度 $O(max(M,N))$：其中 $M$，$N$ 为 $2$ 数字长度，按位遍历一遍数字（以较长的数字为准）；
- 空间复杂度 $O(1)$：指针与变量使用常数大小空间。

<,,,,,,,>

代码：

Python

class Solution:
    def addStrings(self, num1: str, num2: str) -> str:
        res = ""
        i, j, carry = len(num1) - 1, len(num2) - 1, 0
        while i >= 0 or j >= 0:
            n1 = int(num1[i]) if i >= 0 else 0
            n2 = int(num2[j]) if j >= 0 else 0
            tmp = n1 + n2 + carry
            carry = tmp // 10
            res = str(tmp % 10) + res
            i, j = i - 1, j - 1
        return "1" + res if carry else res

Java

class Solution {
    public String addStrings(String num1, String num2) {
        StringBuilder res = new StringBuilder("");
        int i = num1.length() - 1, j = num2.length() - 1, carry = 0;
        while(i >= 0 || j >= 0){
            int n1 = i >= 0 ? num1.charAt(i) - '0' : 0;
            int n2 = j >= 0 ? num2.charAt(j) - '0' : 0;
            int tmp = n1 + n2 + carry;
            carry = tmp / 10;
            res.append(tmp % 10);
            i--; j--;
        }
        if(carry == 1) res.append(1);
        return res.reverse().toString();
    }
}

解题思路： ​

代码： ​

解题思路：

代码：