自律小屋 | 自律小屋

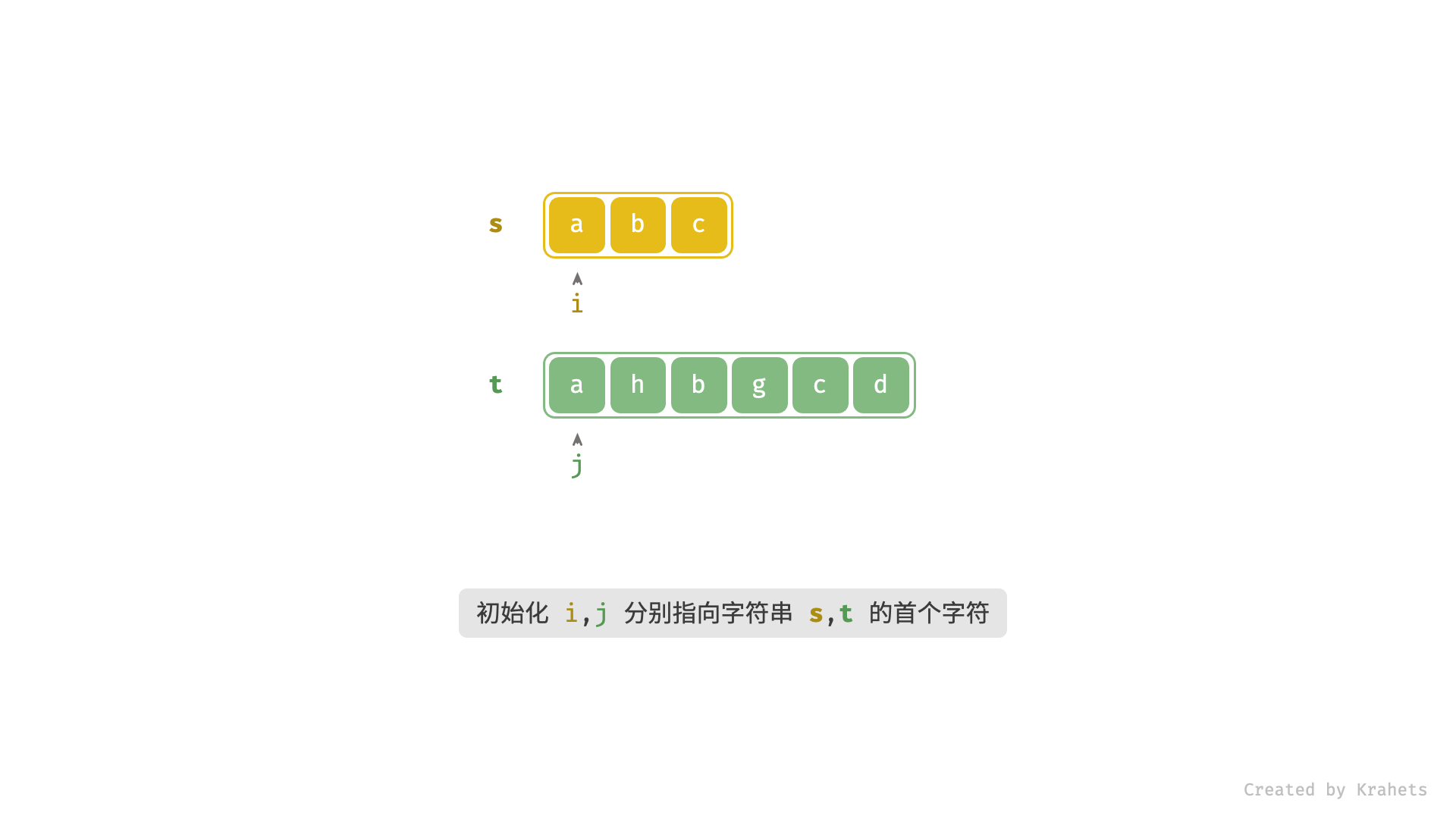
解题思路

设置双指针 i , j 分别指向字符串 s , t 的首个字符，遍历字符串 t ：

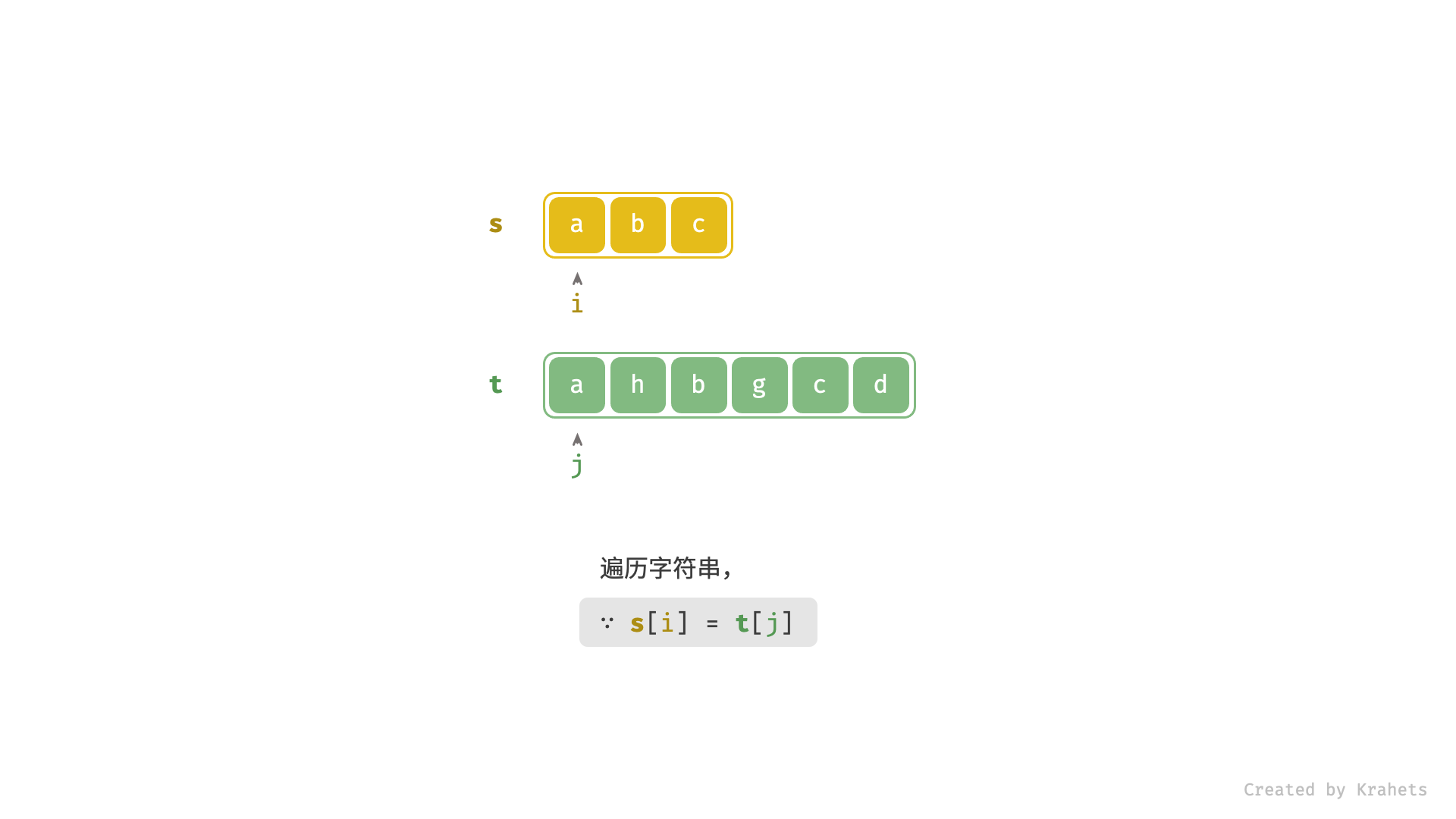
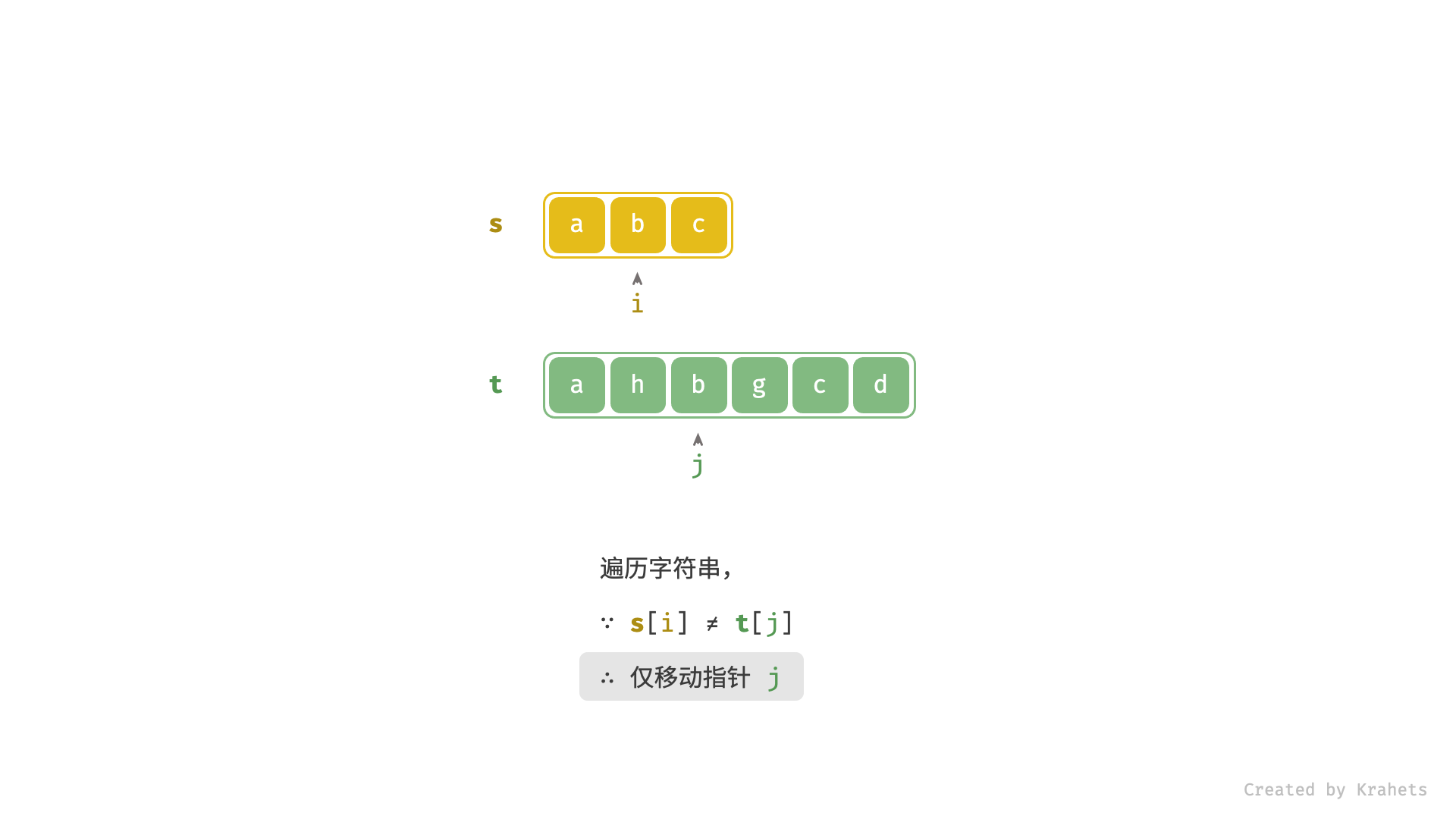
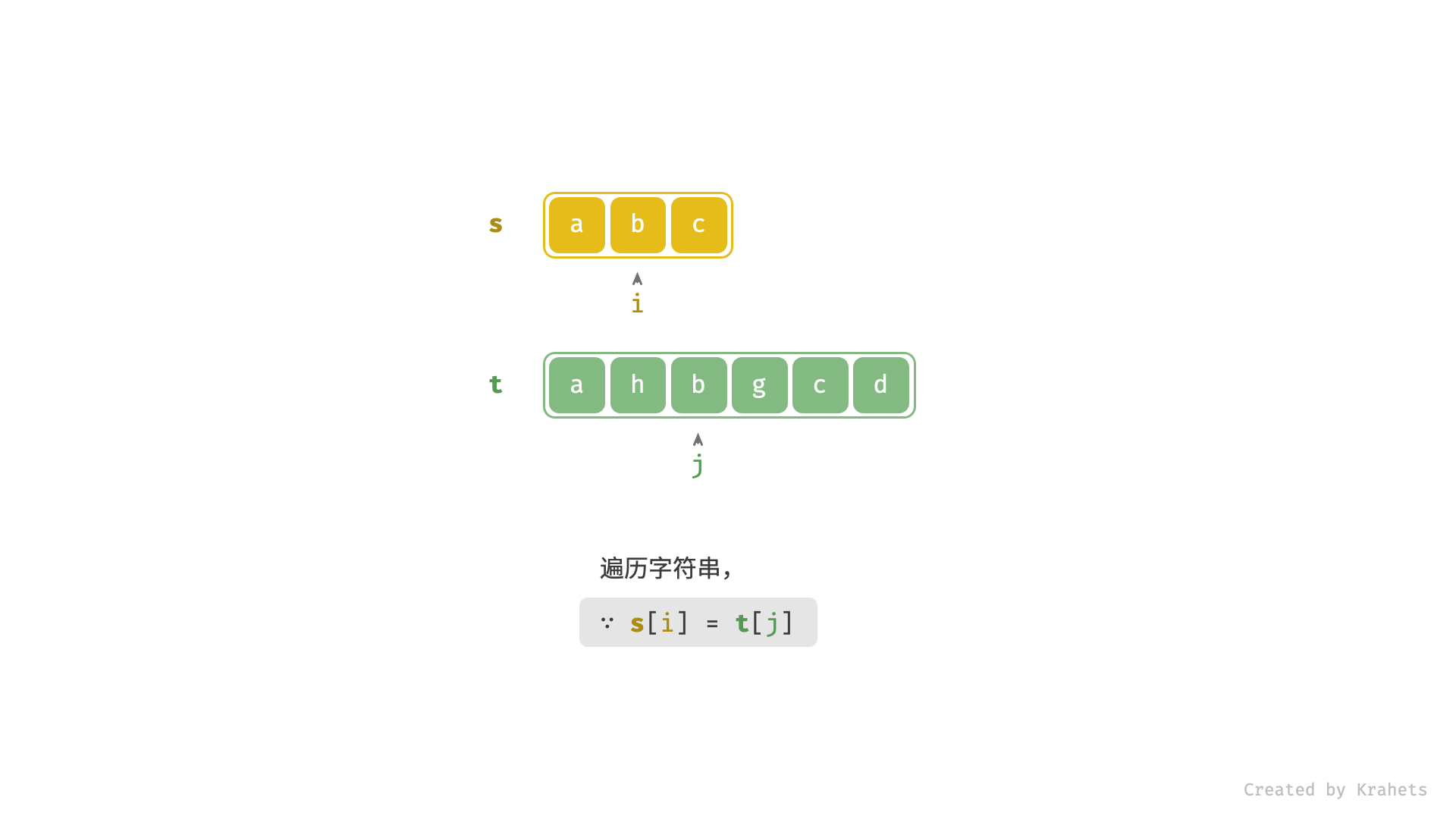
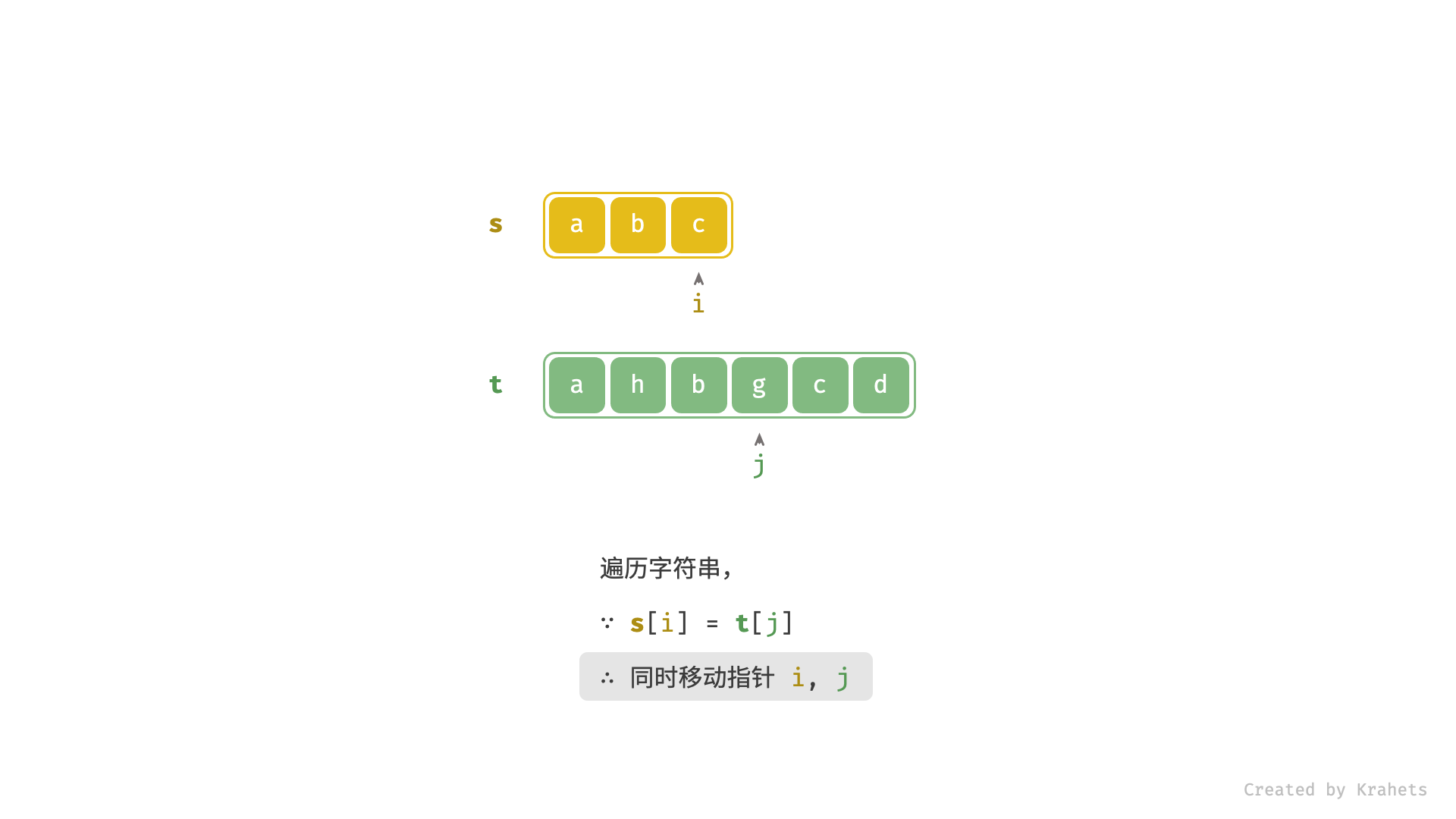
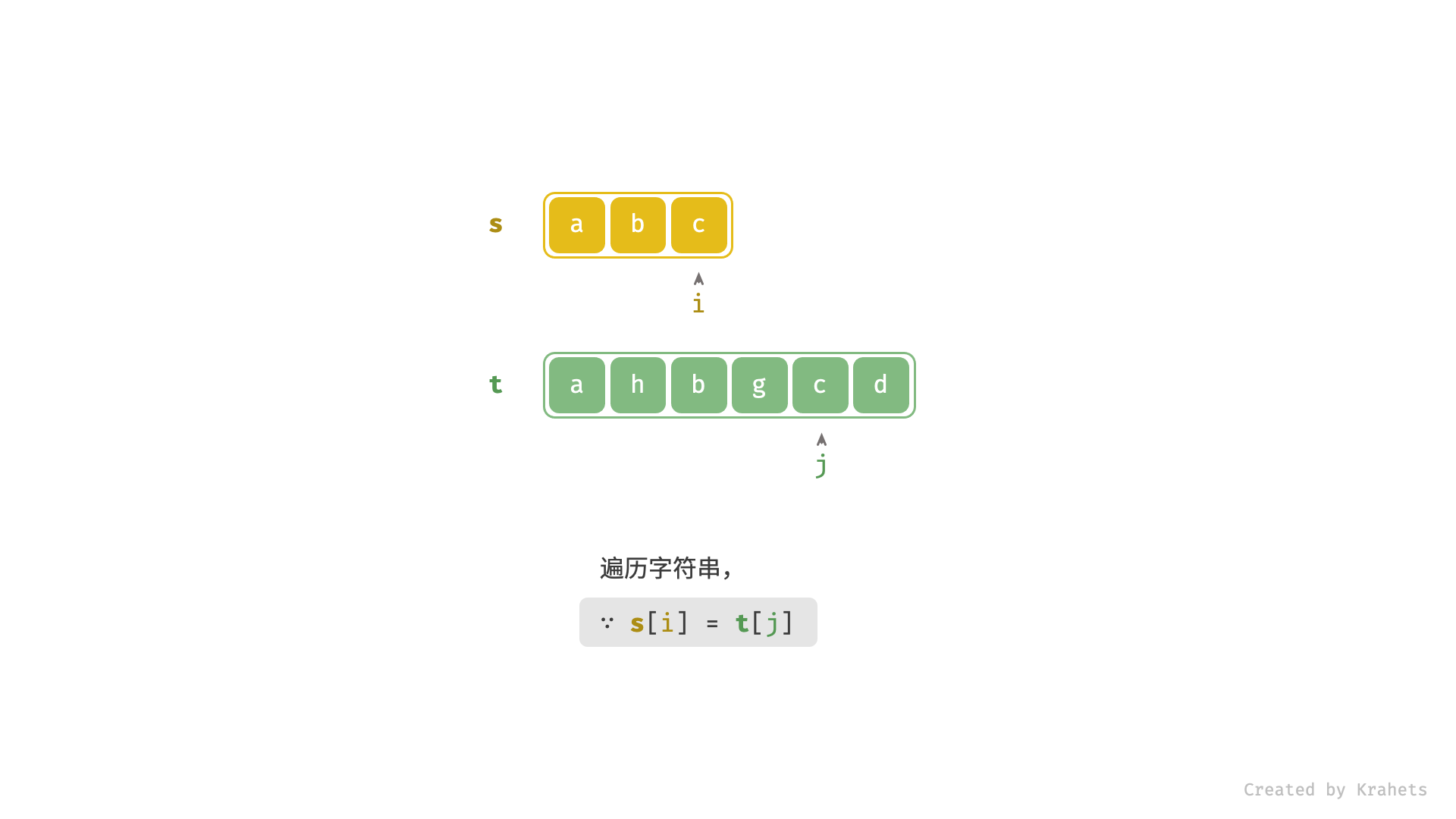
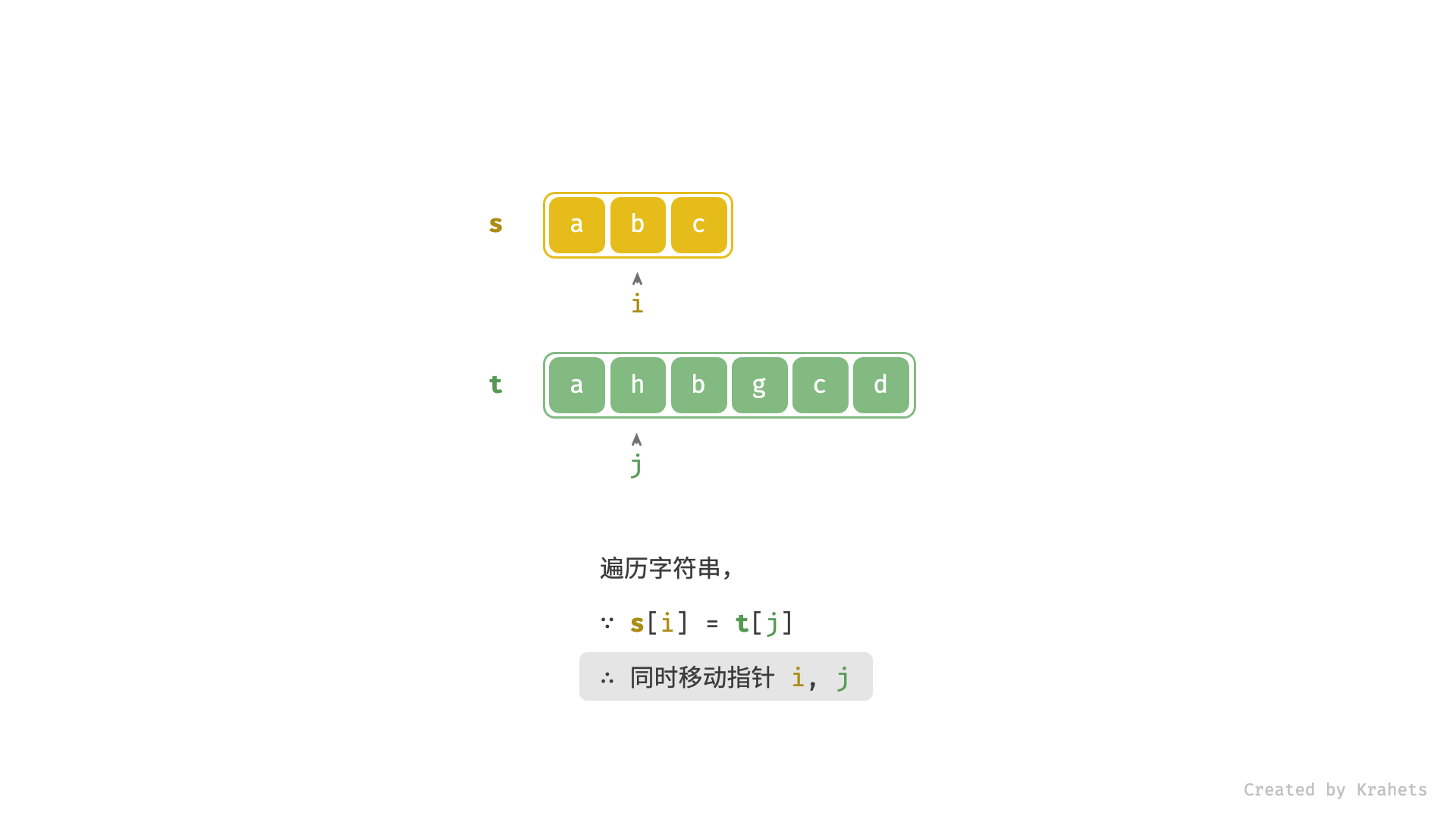
当 s[i] == t[j] 时，代表匹配成功，此时同时 i++ , j++ ；
- 进而，若 i 已走过 s 尾部，代表 s 是 t 的子序列，此时应提前返回 true ；
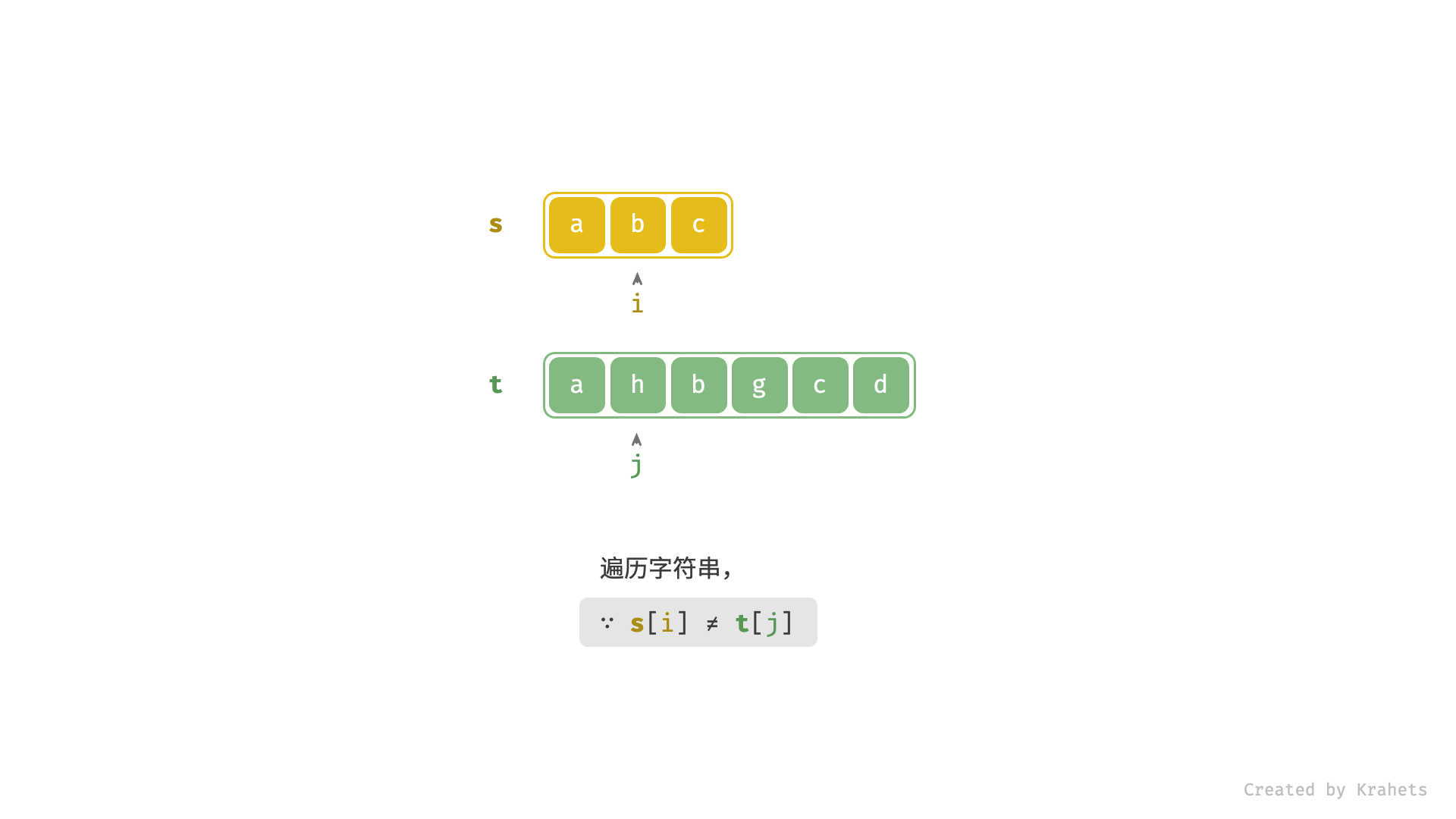
当 s[i] != t[j] 时，代表匹配失败，此时仅 j++ ；

若遍历完字符串 t 后，字符串 s 仍未遍历完，代表 s 不是 t 的子序列，此时返回 false 。

<,,,,,,,,,,>

上为静态图，下为动态图，内容一致。

代码

Python

class Solution:
    def isSubsequence(self, s: str, t: str) -> bool:
        if not s: return True
        i = 0
        for c in t:
            if s[i] == c:
                i += 1
                # 若已经遍历完 s ，则提前返回 true
                if i == len(s):
                    return True
        return False

Java

class Solution {
    public boolean isSubsequence(String s, String t) {
        if (s.length() == 0) return true;
        for (int i = 0, j = 0; j < t.length(); j++) {
            if (s.charAt(i) == t.charAt(j)) {
                // 若已经遍历完 s ，则提前返回 true
                if (++i == s.length())
                    return true;
            }
        }
        return false;
    }
}

C++

class Solution {
public:
    bool isSubsequence(string s, string t) {
        if (s.size() == 0) return true;
        for (int i = 0, j = 0; j < t.size(); j++) {
            if (s[i] == t[j]) {
                // 若已经遍历完 s ，则提前返回 true
                if (++i == s.size())
                    return true;
            }
        }
        return false;
    }
};

复杂度分析

时间复杂度 $O(N)$ ： 其中 $N$ 为字符串 t 的长度。最差情况下需完整遍历 t 。
空间复杂度 $O(1)$ ： i , j 变量使用常数大小空间。

解题思路 ​

代码 ​

复杂度分析 ​

解题思路

代码

复杂度分析