解题思路： ​

本文介绍三种时间复杂度为 $O(N)$ 的解法，其中只有方法三完全满足题目要求，其中：

• 方法一使用了哈希表额外空间；
• 方法二需要修改原数组 nums ；

方法一：哈希表 ​

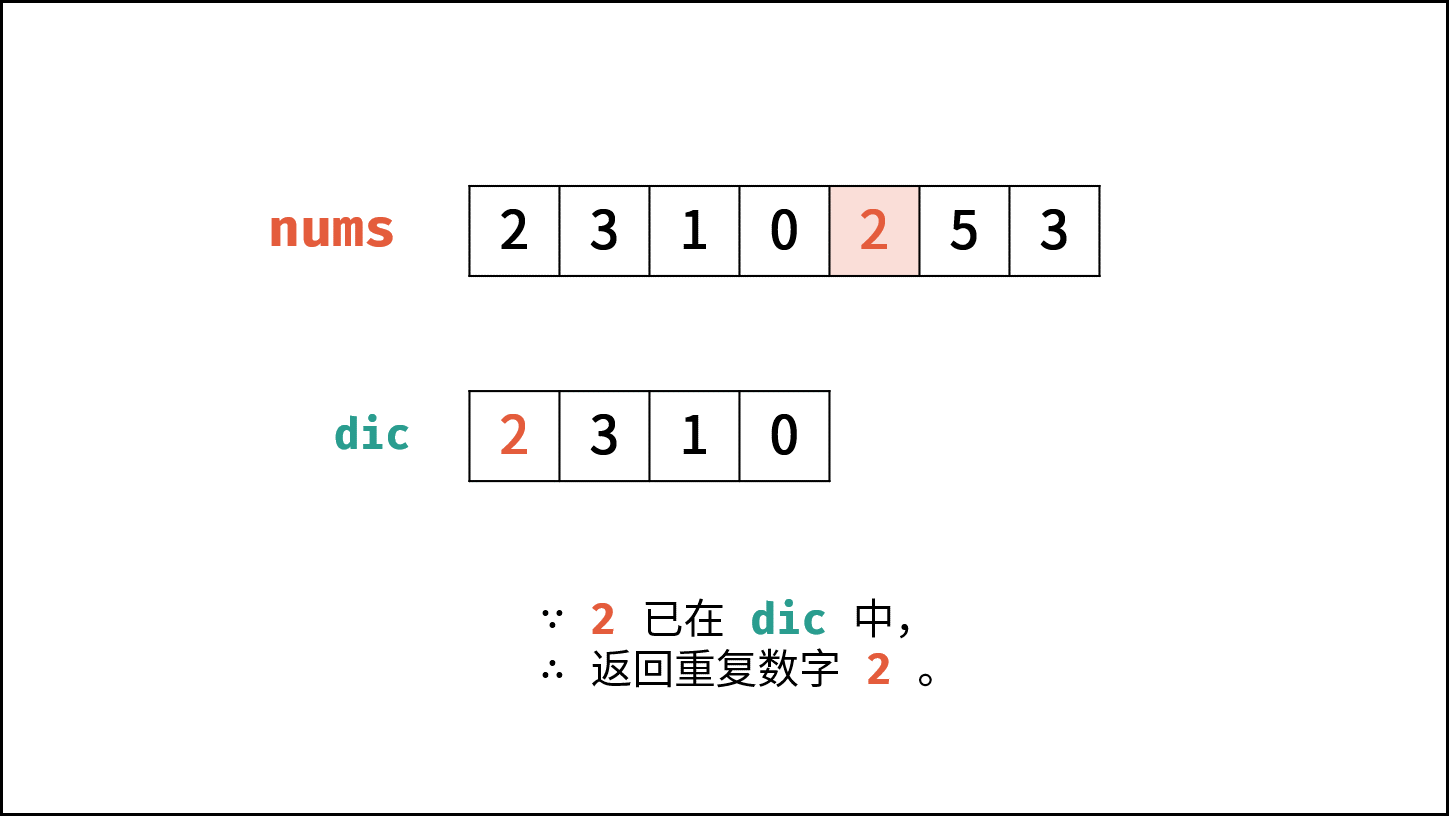
利用数据结构特点，容易想到使用哈希表（Set）记录数组的各个数字，当查找到重复数字则直接返回。

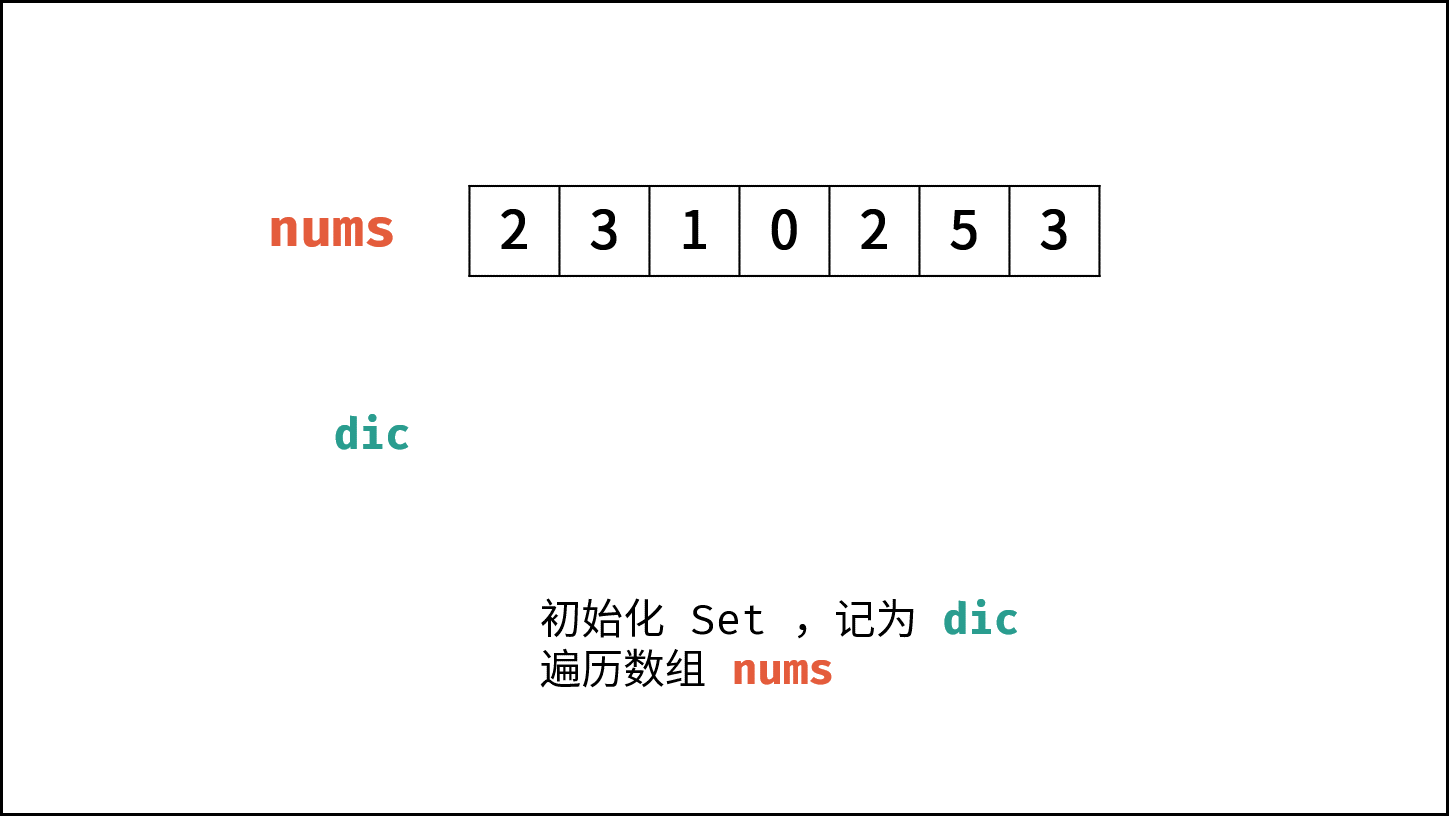
算法流程： ​

1. 初始化： 新建 HashSet ，记为 $hmap$ 。
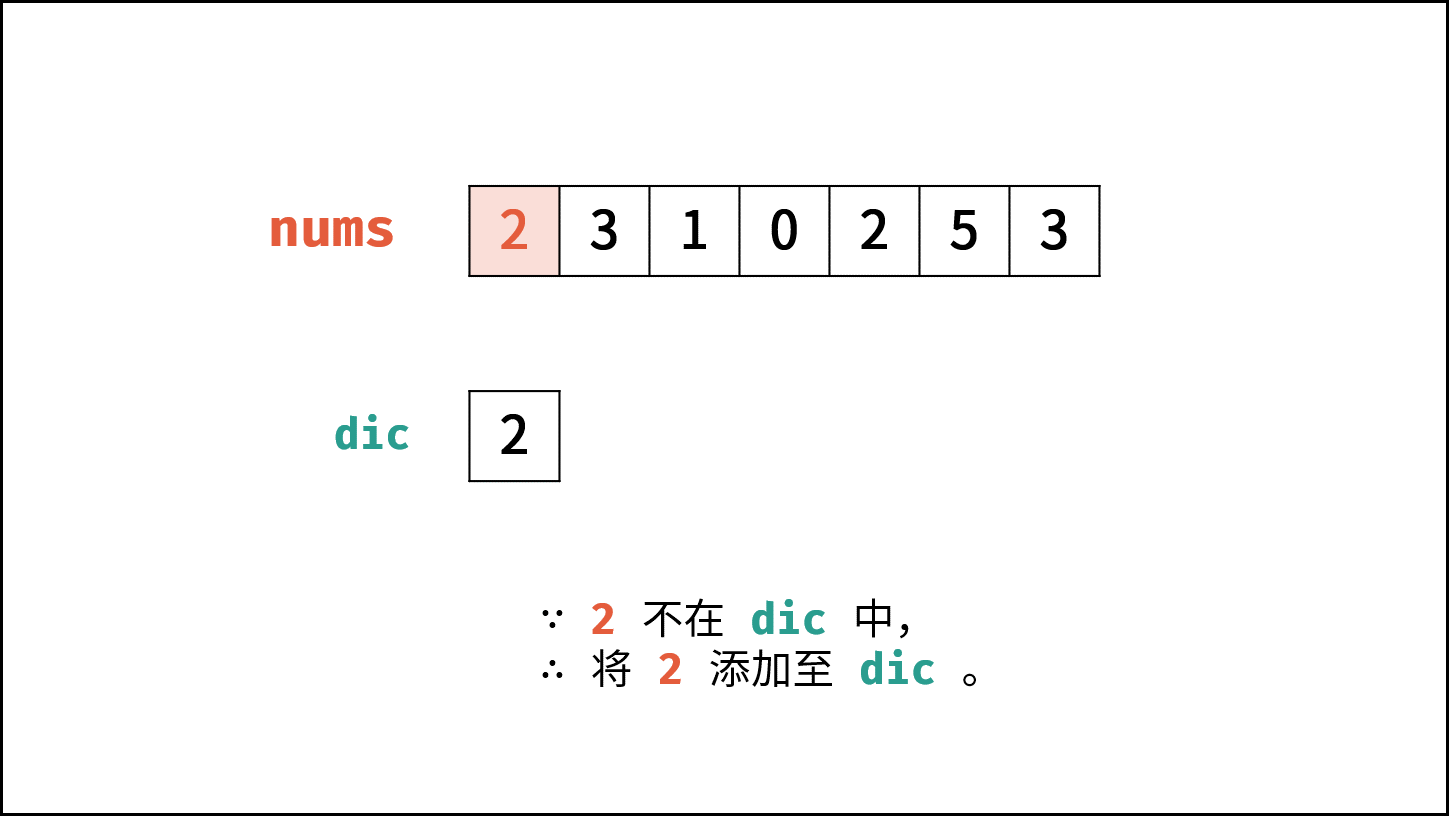
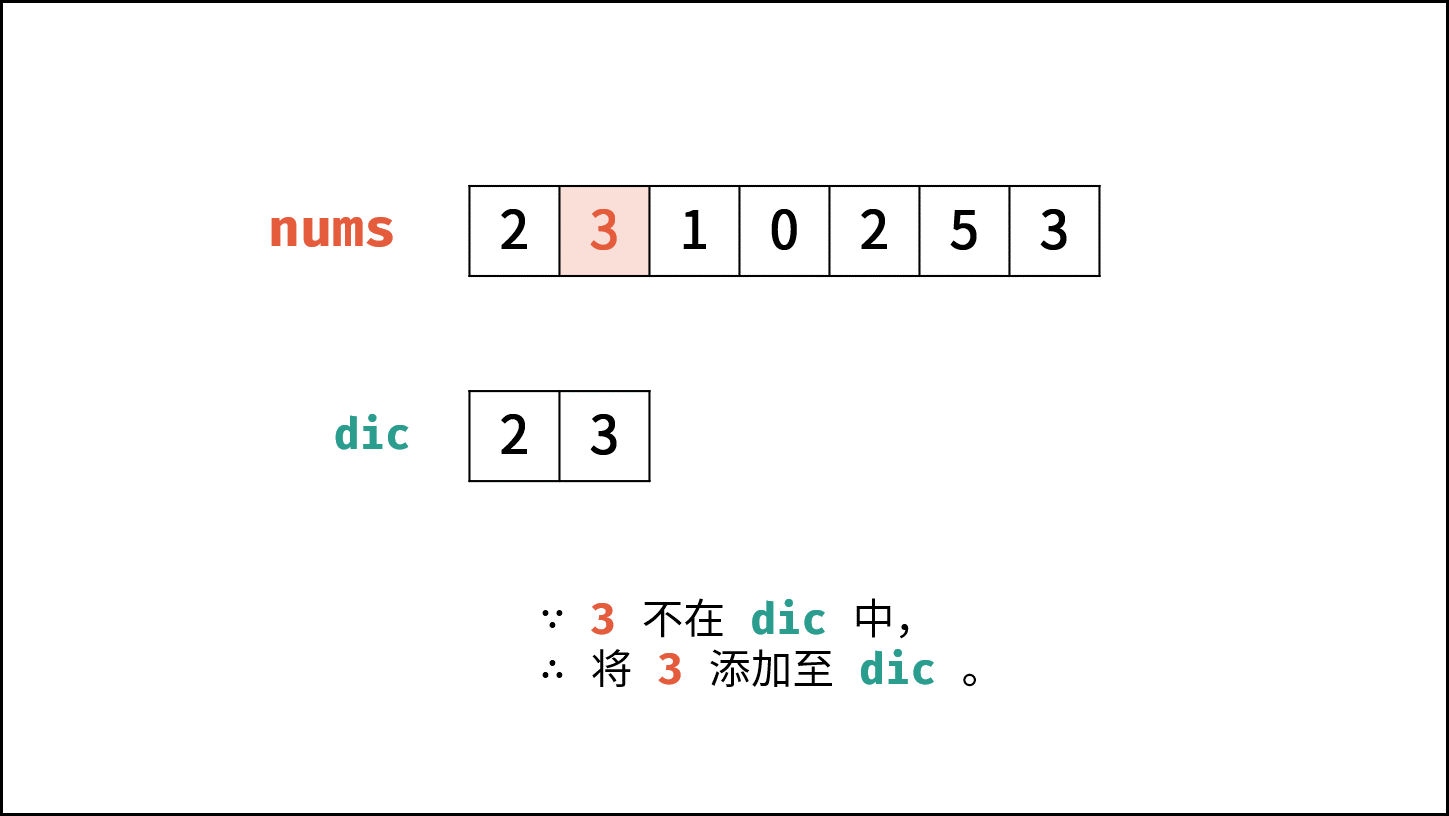
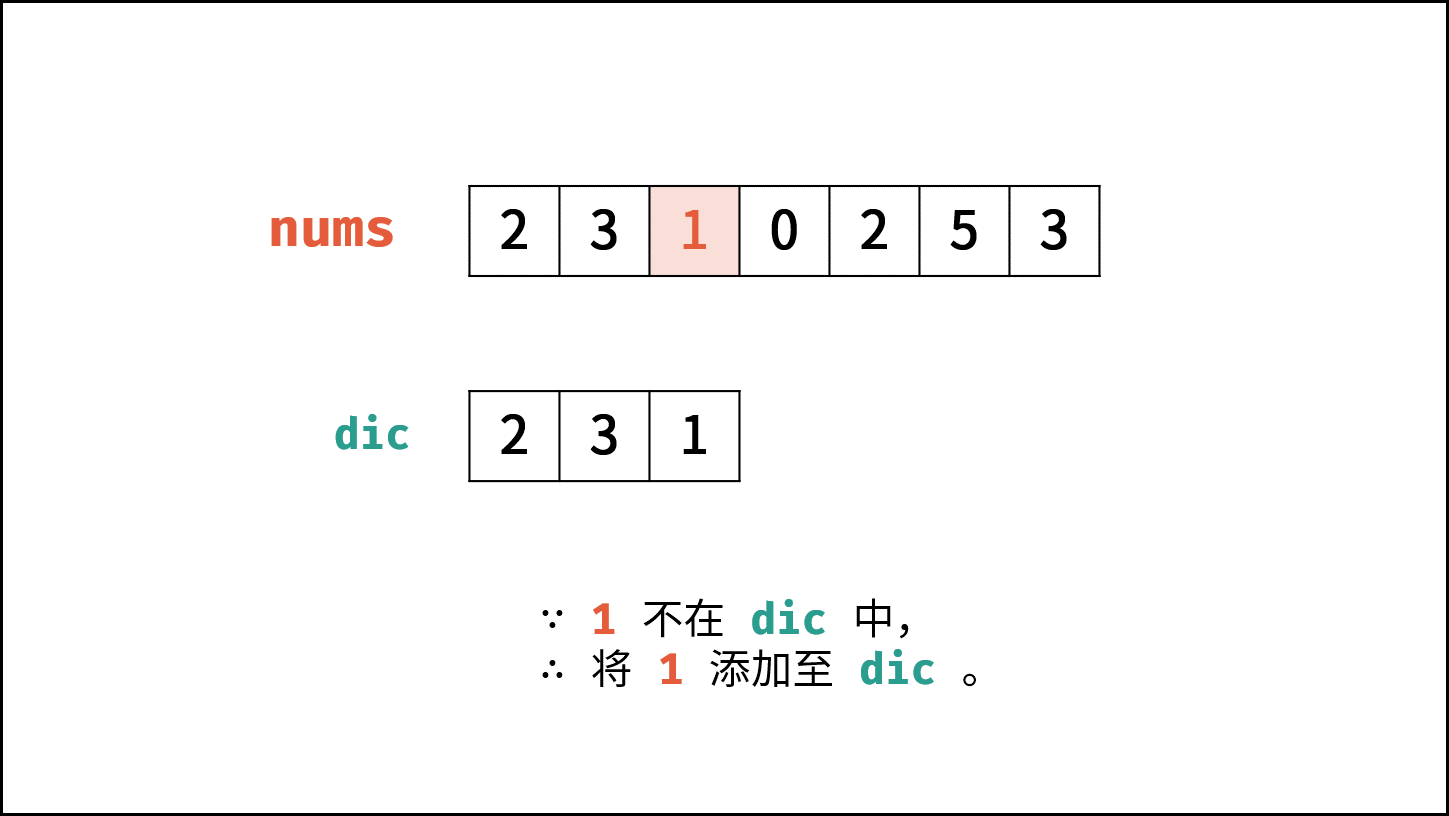
2. 遍历数组 $nums$ 中的每个数字 $num$ ：
   1. 当 $num$ 在 $hmap$ 中，说明重复，直接返回 $num$ 。
   2. 将 $num$ 添加至 $hmap$ 中。
3. 返回 $-1$ 。本题中一定有重复数字，因此这里返回多少都可以。

<,,,,,>

代码： ​

class Solution:
    def findDuplicate(self, nums: List[int]) -> int:
        hmap = set()
        for num in nums:
            if num in hmap: return num
            hmap.add(num)
        return -1

class Solution {
    public int findDuplicate(int[] nums) {
        Set<Integer> hmap = new HashSet<>();
        for(int num : nums) {
            if(hmap.contains(num)) return num;
            hmap.add(num);
        }
        return -1;
    }
}

class Solution {
public:
    int findDuplicate(vector<int>& nums) {
        unordered_map<int, bool> map;
        for(int num : nums) {
            if(map[num]) return num;
            map[num] = true;
        }
        return -1;
    }
};

复杂度分析： ​

• 时间复杂度 $O(N)$ ： 遍历数组使用 $O(N)$ ，HashSet 添加与查找元素皆为 $O(1)$ 。
• 空间复杂度 $O(N)$ ： HashSet 占用 $O(N)$ 大小的额外空间。

方法二：原地交换 ​

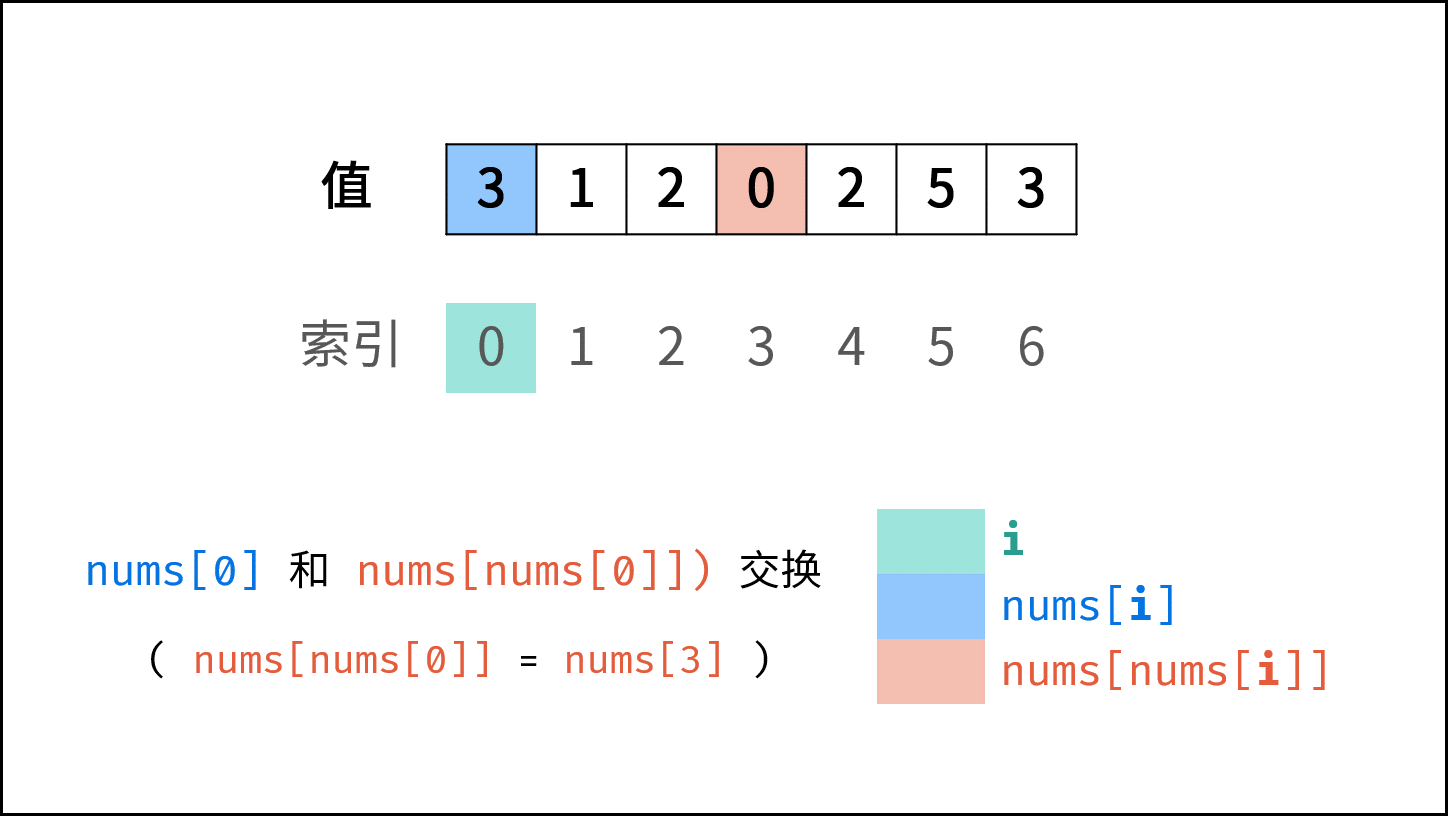
题目说明尚未被充分使用，即 在一个长度为 n 的数组 nums 里的所有数字都在 0 ~ n-1 的范围内 。 此说明含义：数组元素的 索引 和 值 是 一对多 的关系。 因此，可遍历数组并通过交换操作，使元素的 索引 与 值 一一对应（即 $nums[i] = i$ ）。因而，就能通过索引映射对应的值，起到与字典等价的作用。

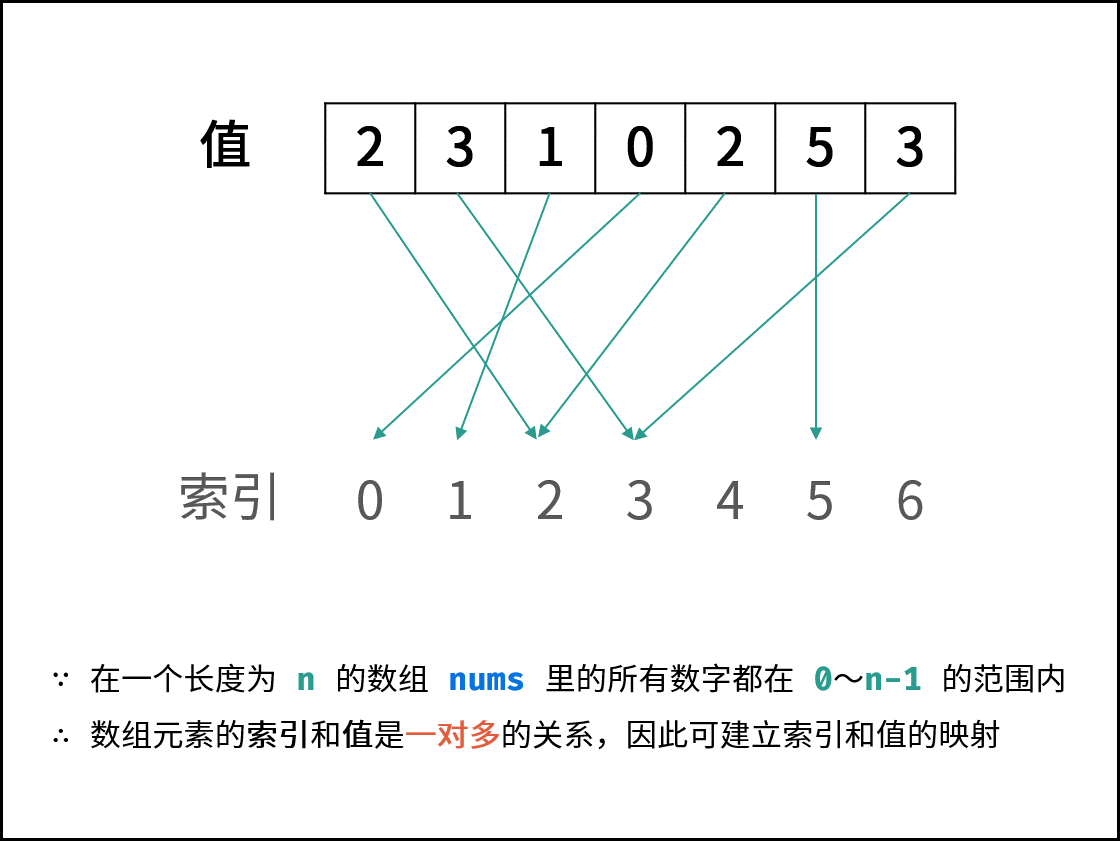
遍历中，第一次遇到数字 $x$ 时，将其交换至索引 $x$ 处；而当第二次遇到数字 $x$ 时，一定有 $nums[x] = x$ ，此时即可得到一组重复数字。

算法流程： ​

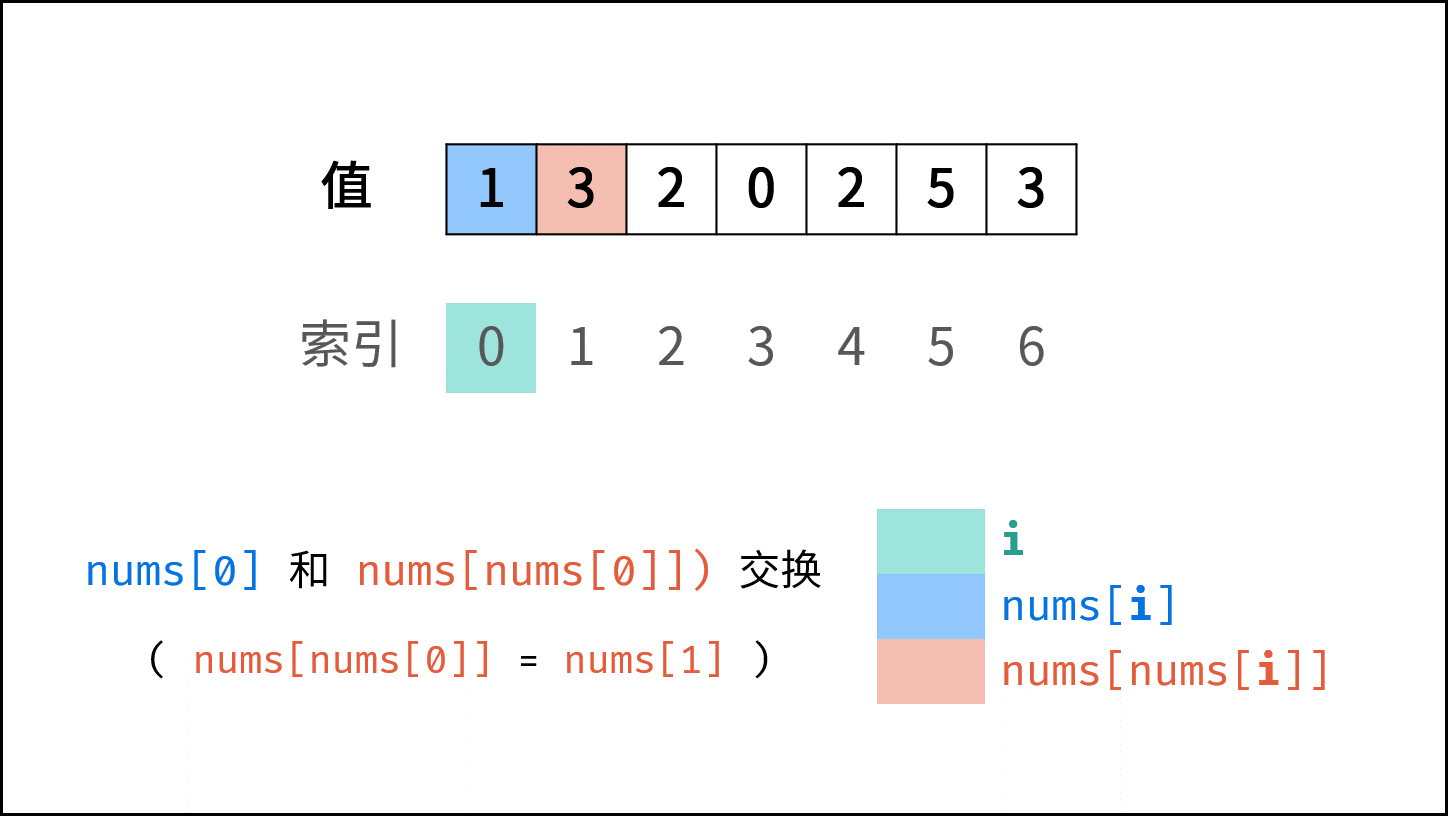
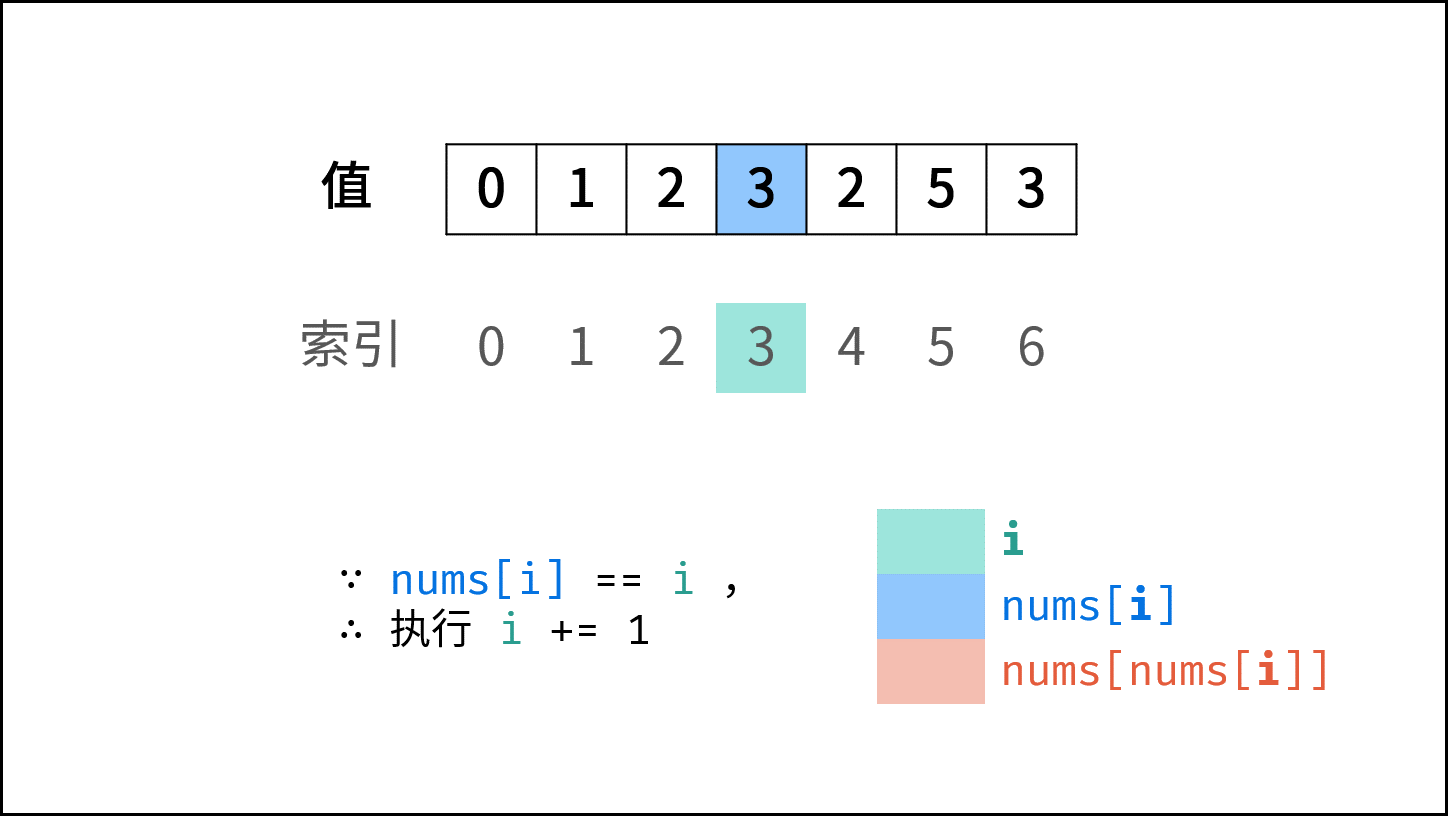
1. 遍历数组 $nums$ ，设索引初始值为 $i = 0$ :

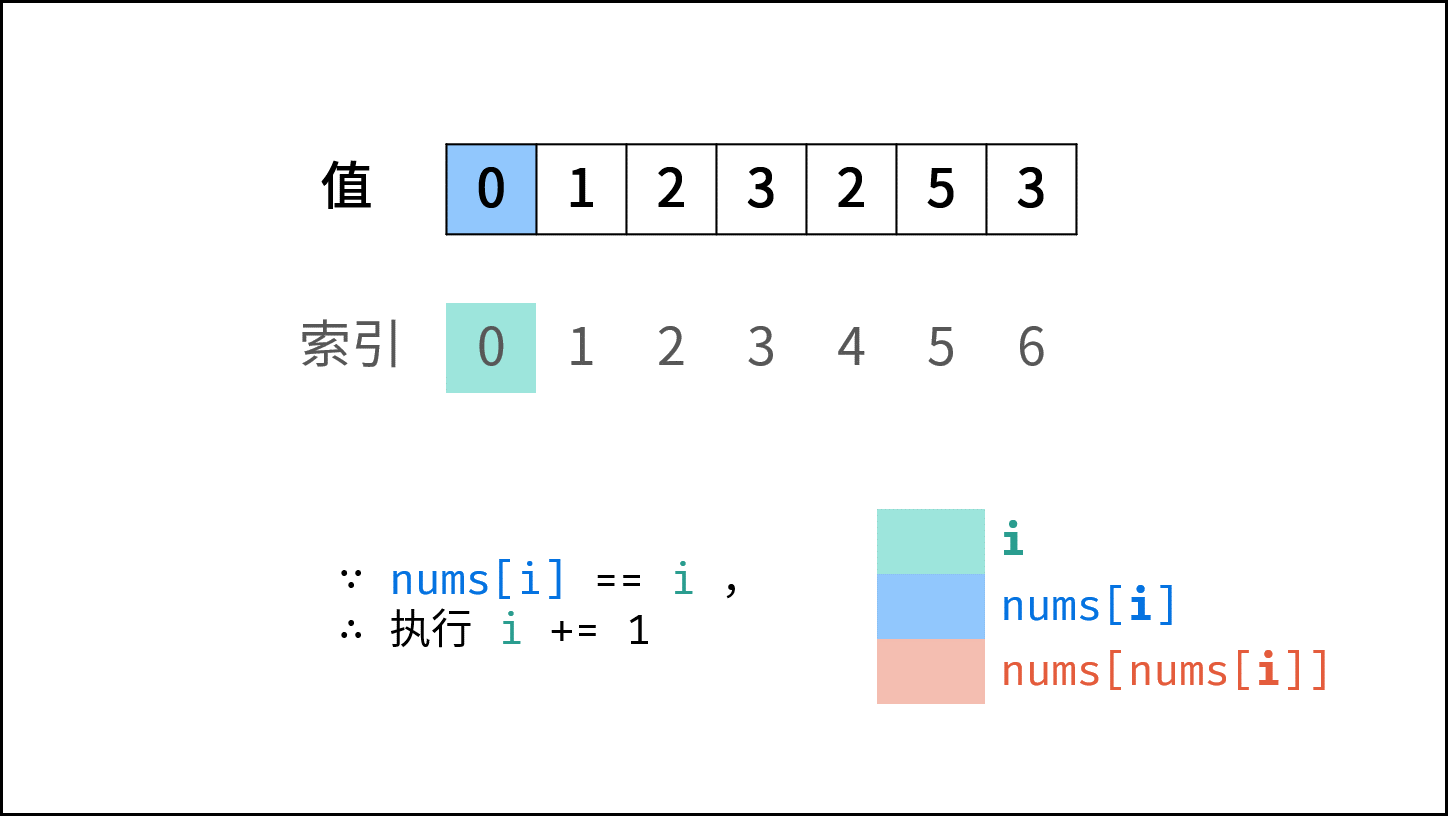
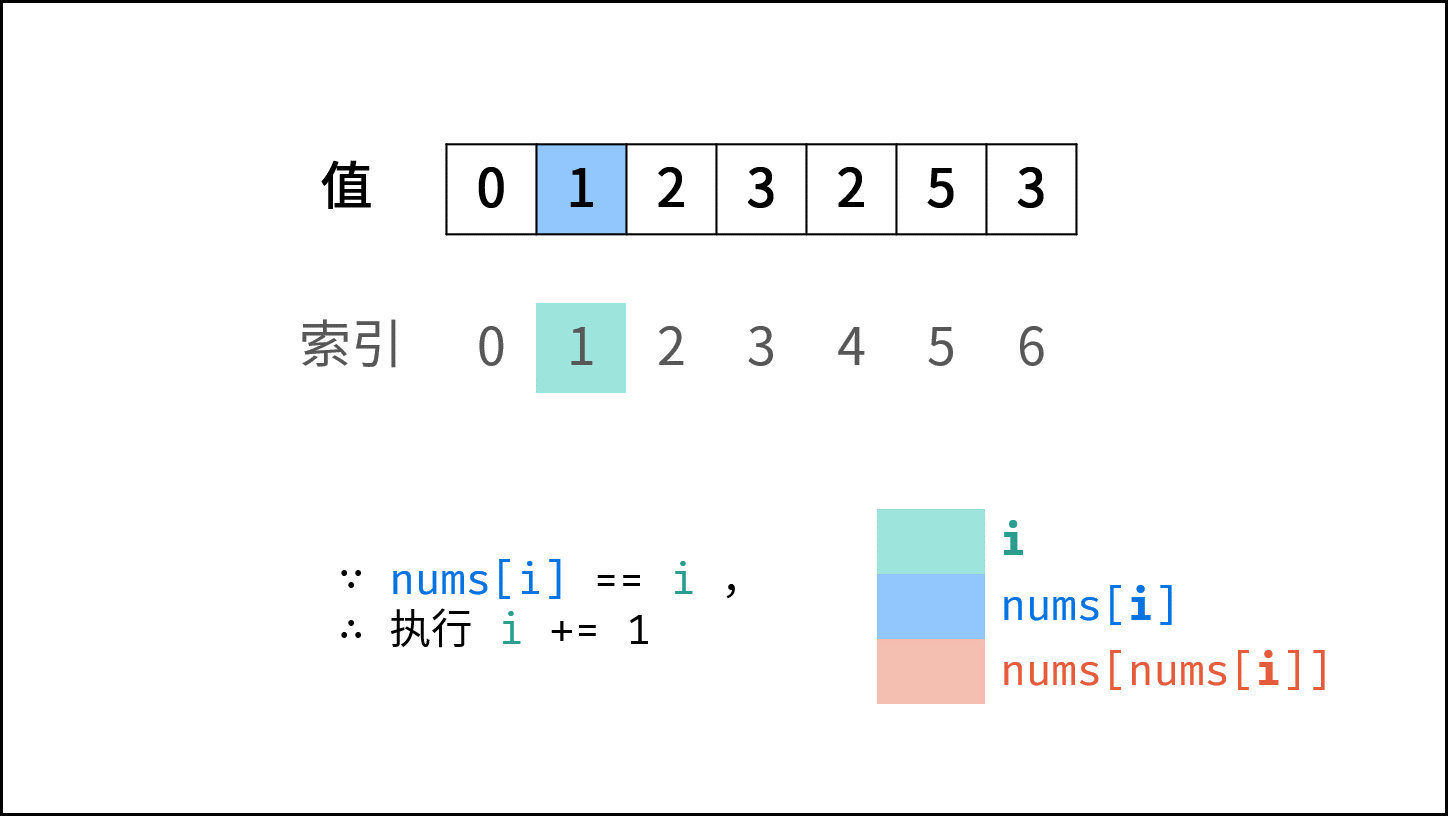
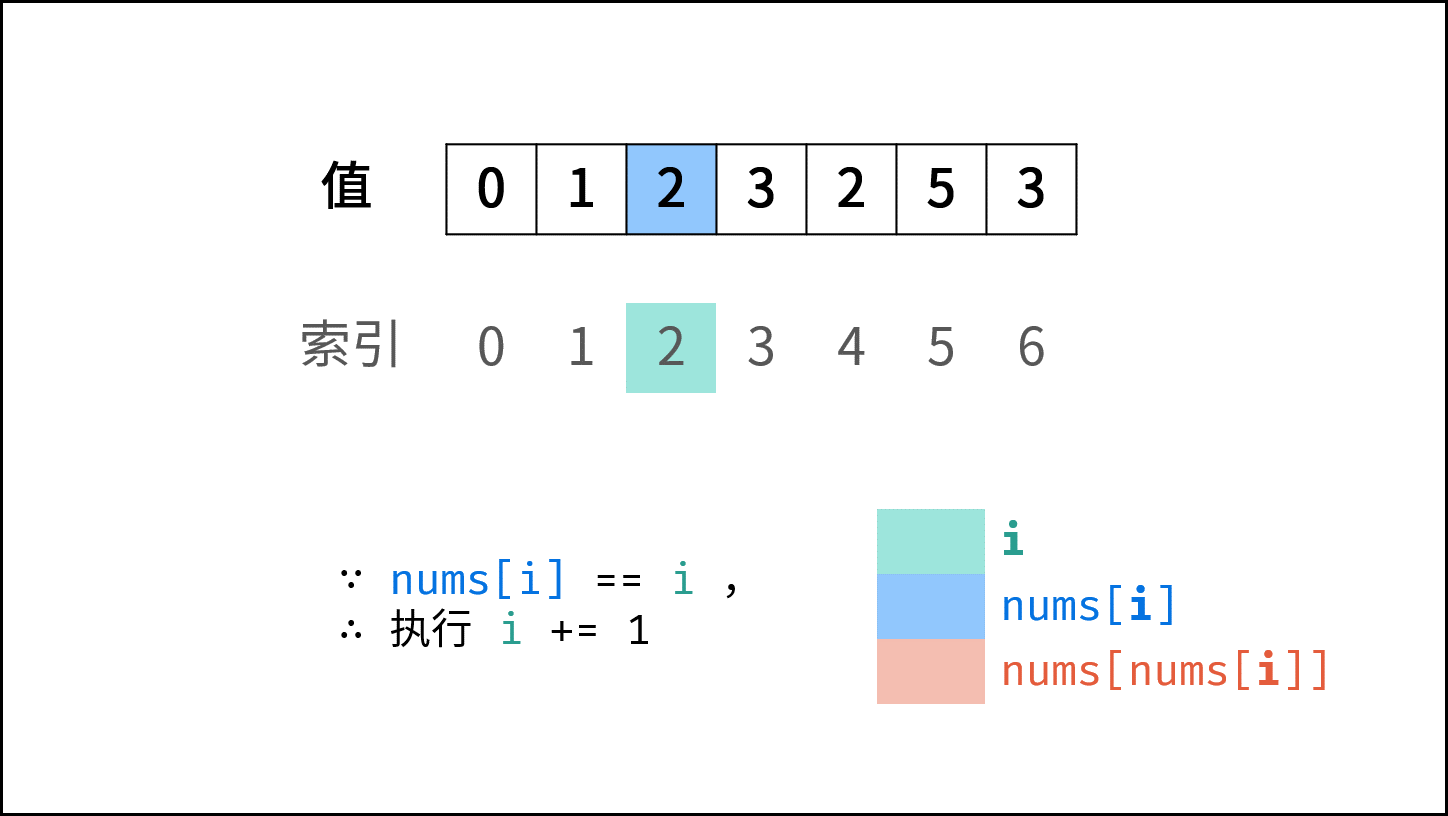
   1. 若 $nums[i] = i$ ： 说明此数字已在对应索引位置，无需交换，因此跳过。
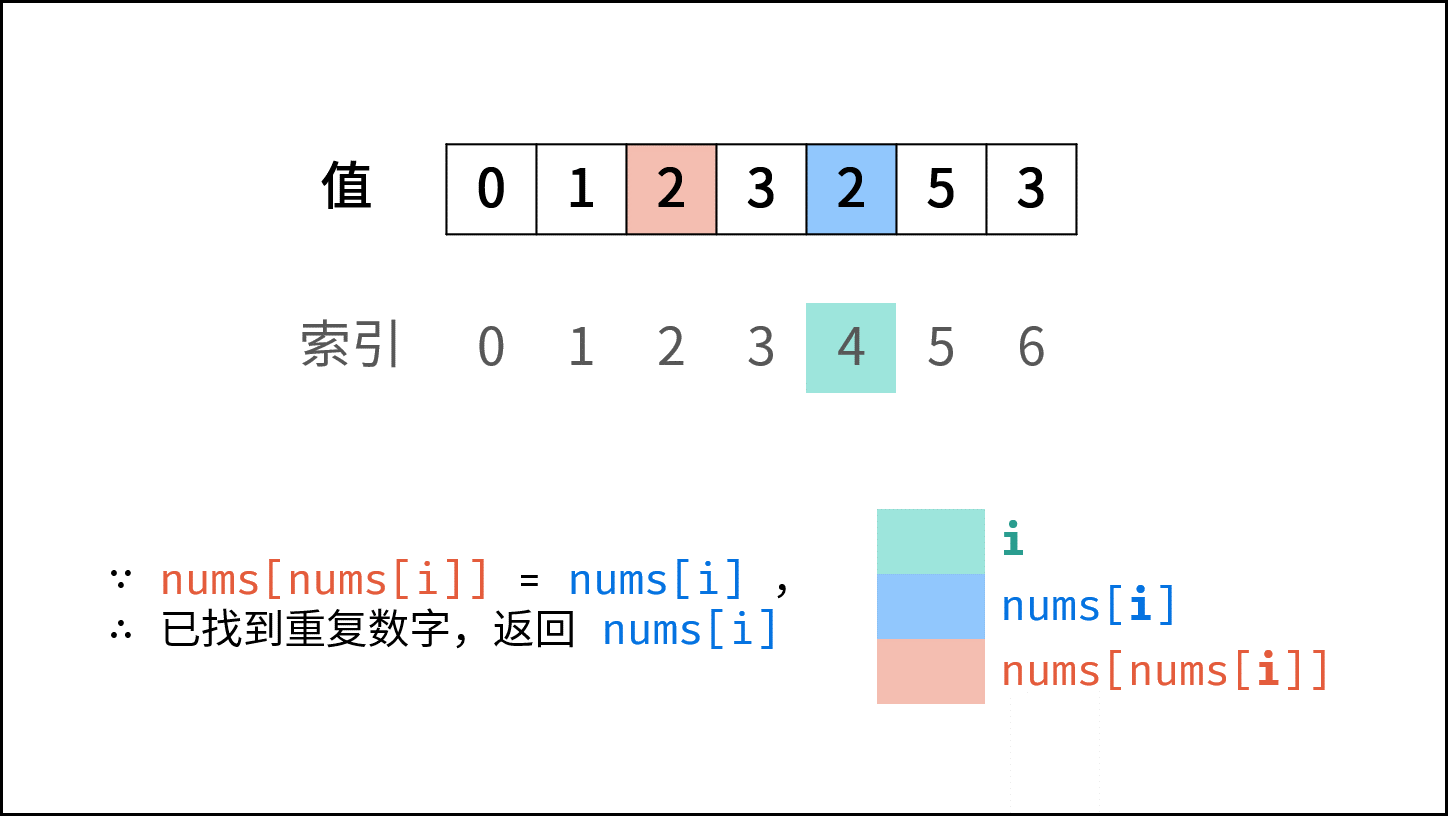
   2. 若 $nums[nums[i]] = nums[i]$ ： 代表索引 $nums[i]$ 处和索引 $i$ 处的元素值都为 $nums[i]$ ，即找到一组重复值，返回此值 $nums[i]$ 。
   3. 否则： 交换索引为 $i$ 和 $nums[i]$ 的元素值，将此数字交换至对应索引位置。

2. 若遍历完毕尚未返回，则返回 $-1$ 。

<,,,,,,,,>

代码： ​

Python 中， $a, b = c, d$ 操作的原理是先暂存元组 $(c, d)$ ，然后 “按左右顺序” 赋值给 a 和 b 。 因此，若写为 $nums[i], nums[nums[i]] = nums[nums[i]], nums[i]$ ，则 $nums[i]$ 会先被赋值，之后 $nums[nums[i]]$ 指向的元素则会出错。

class Solution:
    def findDuplicate(self, nums: List[int]) -> int:
        i = 0
        while i < len(nums):
            if nums[i] == i:
                i += 1
                continue
            if nums[nums[i]] == nums[i]: return nums[i]
            nums[nums[i]], nums[i] = nums[i], nums[nums[i]]
        return -1

class Solution {
    public int findDuplicate(int[] nums) {
        int i = 0;
        while(i < nums.length) {
            if(nums[i] == i) {
                i++;
                continue;
            }
            if(nums[nums[i]] == nums[i]) return nums[i];
            int tmp = nums[i];
            nums[i] = nums[tmp];
            nums[tmp] = tmp;
        }
        return -1;
    }
}

class Solution {
public:
    int findDuplicate(vector<int>& nums) {
        int i = 0;
        while(i < nums.size()) {
            if(nums[i] == i) {
                i++;
                continue;
            }
            if(nums[nums[i]] == nums[i])
                return nums[i];
            swap(nums[i],nums[nums[i]]);
        }
        return -1;
    }
};

复杂度分析： ​

• 时间复杂度 $O(N)$ ： 遍历数组使用 $O(N)$ ，每轮遍历的判断和交换操作使用 $O(1)$ 。
• 空间复杂度 $O(1)$ ： 使用常数复杂度的额外空间。

方法三：环形链表 ​

根据题意，数组索引和元素的取值范围 $\in [1, n]$ 。我们考虑建立一个 $n$ 个节点的链表：

• $n$ 个节点的值：$1$ , $2$ , $\cdots$ , $n$ ；
• 对于每个节点 $i$ ，其 next 引用指向节点 $nums[i]$ 。

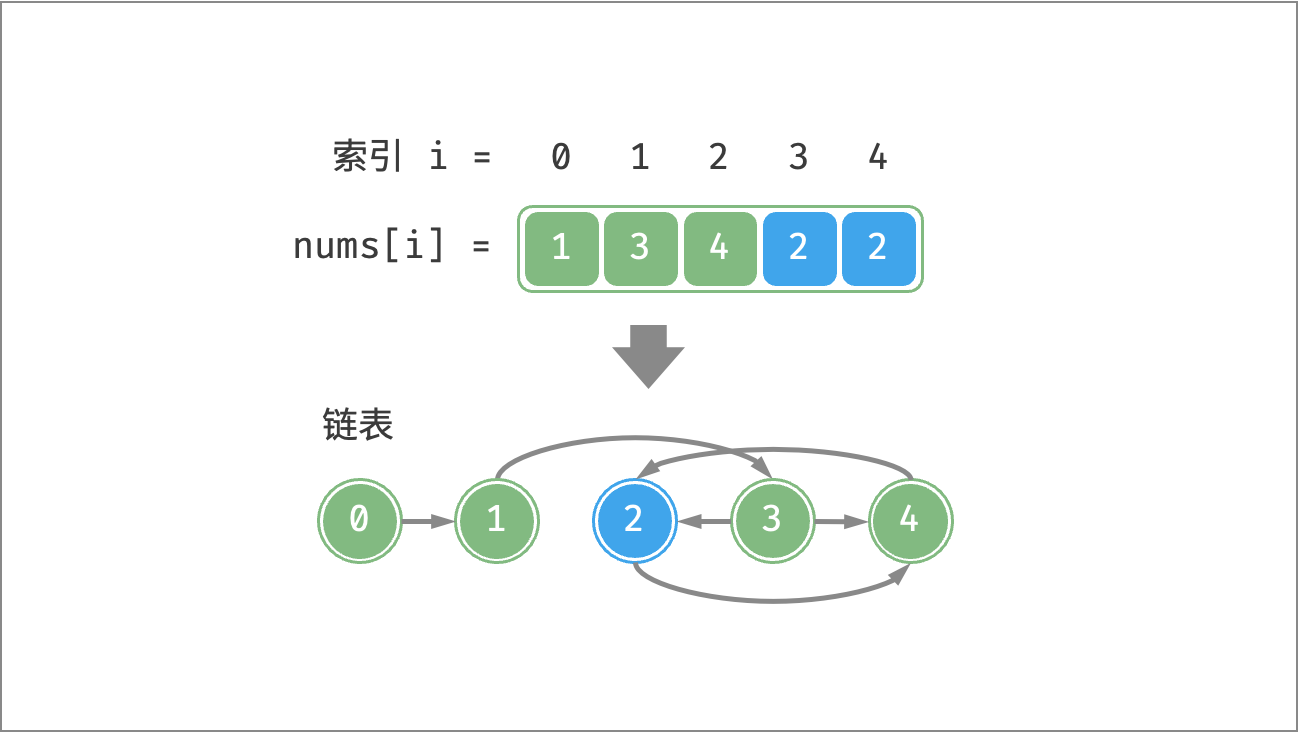
假设重复元素为 $x$ ，那么在这个链表中，一定同时有两条边指向节点 $x$ 。例如在上图中，有两条边都指向节点 $2$ 。因此可得到推论：此链表中一定存在环，且节点 $x$ 是环的入口。

换句话说，找出重复元素 $x$ 等价于找出链表中环的入口。这个问题实际上就是环形链表 II，唯一的不同点在于我们需要在数组中进行链表操作。

代码： ​

class Solution:
    def findDuplicate(self, nums: List[int]) -> int:
        def next(i):
            return nums[i]
        slow = fast = 0
        # 第一次相遇
        while True:
            slow = next(slow)
            fast = next(next(fast))
            if slow == fast:
                break
        slow = 0
        # 第二次相遇
        while slow != fast:
            slow = next(slow)
            fast = next(fast)
        return slow

public class Solution {
    private int next(int[] nums, int index) {
        return nums[index];
    }
    public int findDuplicate(int[] nums) {
        int slow = 0;
        int fast = 0;
        // 第一次相遇
        do {
            slow = next(nums, slow);
            fast = next(nums, next(nums, fast));
        } while (slow != fast);
        slow = 0;
        // 第二次相遇
        while (slow != fast) {
            slow = next(nums, slow);
            fast = next(nums, fast);
        }
        return slow;
    }
}

class Solution {
public:
    vector<int> nums;
    int next(int index) {
        // 直接返回当前索引处的值作为下一个索引
        return nums[index];
    }
    int findDuplicate(vector<int>& nums) {
        this->nums = nums;
        int slow = 0;
        int fast = 0;
        // 第一次相遇
        do {
            slow = next(slow);
            fast = next(next(fast));
        } while (slow != fast);
        slow = 0;
        // 第二次相遇
        while (slow != fast) {
            slow = next(slow);
            fast = next(fast);
        }
        return slow;
    }
};

复杂度分析： ​

• 时间复杂度 $O(N)$ ： 遍历链表使用 $O(N)$ 。
• 空间复杂度 $O(1)$ ： 使用常数复杂度的额外空间。